Math 103 Notes and Outlines

Martin Flashman's Courses- Math 103 Spring, 2004
[Tentative] Class Topic Notes and Outlines
Work in Progress- Subject to Change!

Tuesday

Thursday

1-20
Introduction to "Visual Math"

1-22
The Pythagorean Theorem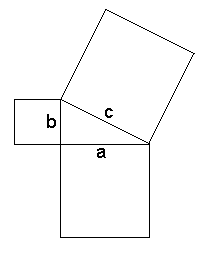

a²+ b²= c²

1-27
Tangrams and Dissection Puzzles

1 -29
Dissection Puzzles &
Scissors Congruent
(Equidecomposable)
Polygons

2-3
Dissection Theorem for Regular Polygons
BeginTilings of the Plane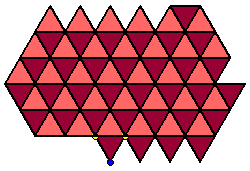

2-5
Regular and Semi- regular Tilings of the Plane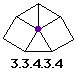

2-10
Finish Semi-Regular Tilings
Symmetries for a Single Polygon
Reflections and Rotations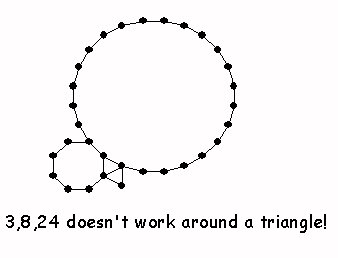

2-12
Symmetries
for a Triangle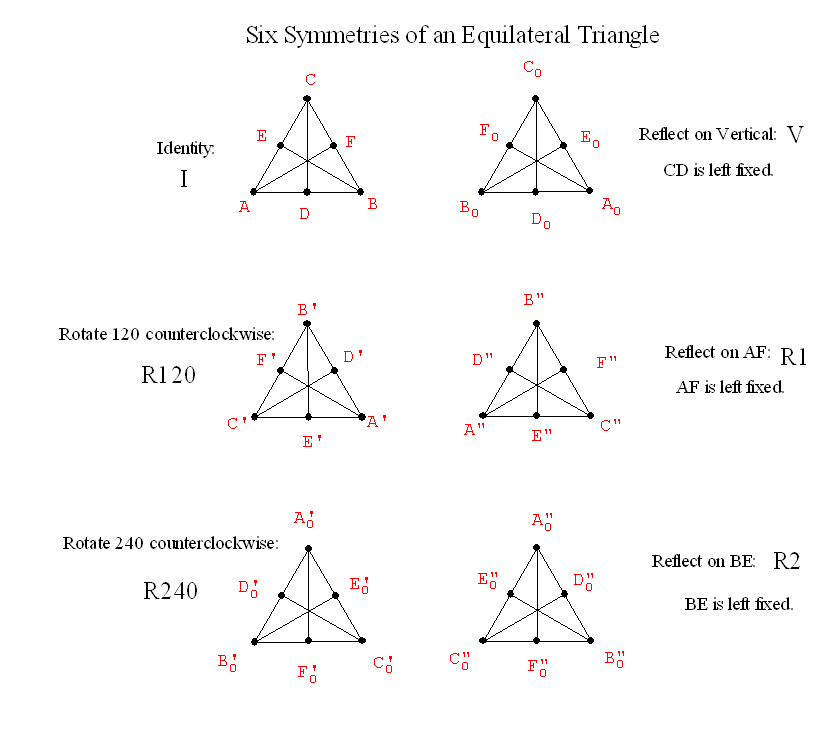
Symmetries of an equilateral triangle.

2-17
Symmetries for a Tiling of a Freize and the Plane
Isometries of the Plane.
...|p|q|p|q|p|q|p|q|p|q|p|...
...|d|b|d|b|d|b|d|b|d|b|d|...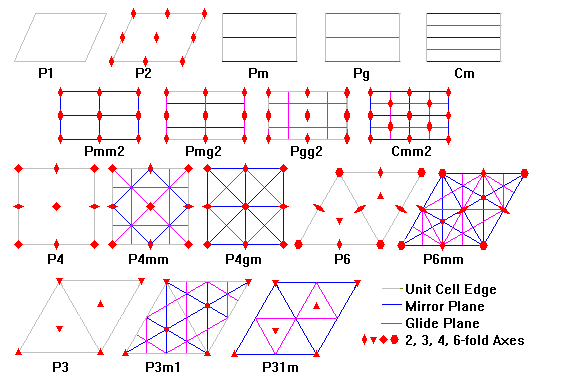

2-19
Isometries in Symmetry Groups
and planar tilings.
Begin Space- Symmetries and Isometries
Rotations and Reflections

2-24
Spatial Objects:
Getting Familiar with The Platonic Solids.

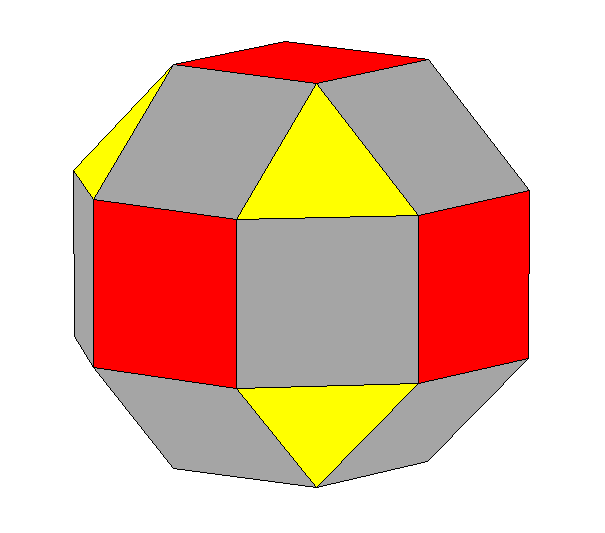
2-26
The Platonic and Archimedean Solids.

Cubeoctahedron

Rhombicubeoctahedron

Icosidodecahedro

3-2
More on Solids. Symmetry. Isometries in Space.

3-4
Connections between Polyhedra. Frameworks. Duality.
Similarity in the plane and space.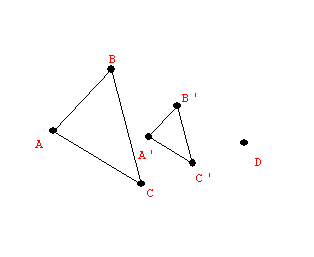

3-9
More on Similarity, Geometric Sequences, and Series

Big

3-11
Space Filling Curves

3-16
Spring Break No Class

3-18
Spring Break No Class

3-23
Encounters with The Fourth Dimension
The Hypercube.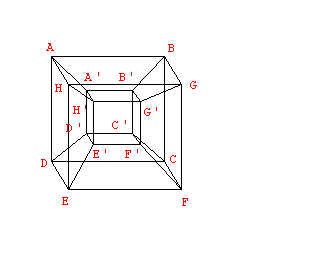

3-25
More on the Hypercube:Coordinates

3-30

Coordinates for the Hypercube and the Tower of Hanoi

*
*
*

*

4-1
Maps and Coordinates for Surfaces
Flatland, The Earth and The Torus.
Begin Topology for curves.

4-6
Counting on curves and in the plane.
Euler's Formula

V+R = E + 2

4-8
Appplications of the Euler Formula
"A Hard Problem"
What's possible and what's impossible!
The Color Problems on the plane, the sphere, and the torus...

4-13
Other Surfaces- The Sphere and the Torus and Beyond:
Adventures on the Mobius Band, the Klein Bottle, and
the Projective Plane?

4-15
More on Surfaces

4-20
More on Surfaces
The Classification of Surfaces
"New" Surfaces

4-22
The Classification of Surfaces
Euler's Characteristic Number
"New" Surfaces
Cones and Conic Sections- Projective Geometry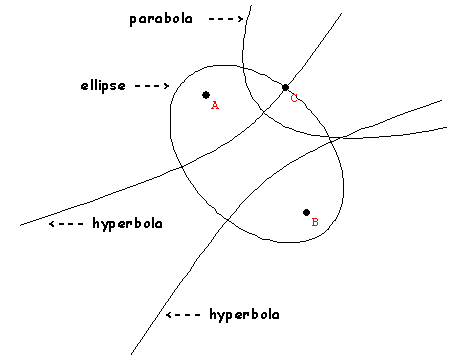

4-27
More on the Conics
Projective Geometry:
An Introduction to Desargues' Theorem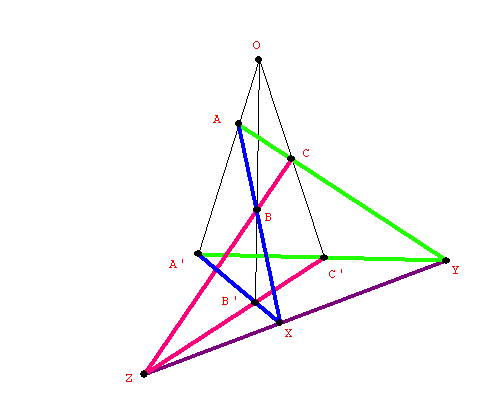

4-29
Perspective and Projective Geometry

Perspective in Space and The Projective Plane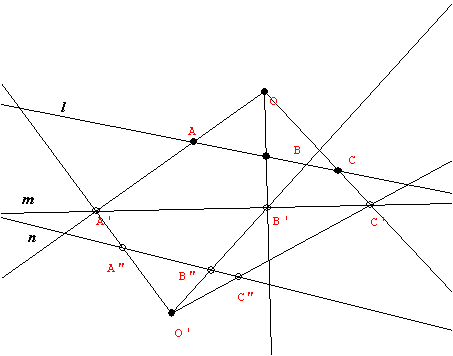

5-4
More about Perspective and the Projective Plane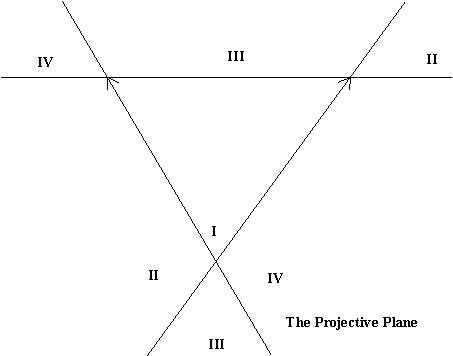

5-6
Other Worlds and Surfaces:
A Non-euclidean Universe.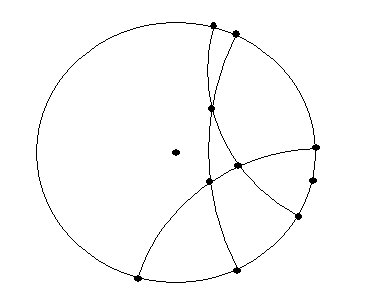

Inventory

Turning a sphere inside out.

Maps and Projective Geometry

Projective Geometry:
Desargues' Theorem ,Duality, Pascal's Theorem and The Conics!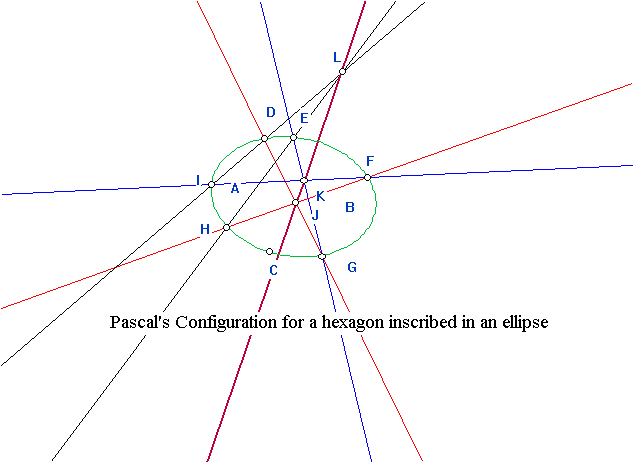

More Duality and Proofs.
What is possible and what is not!
Properties of Curves and Surfaces:
Geometric, projective, and topological.