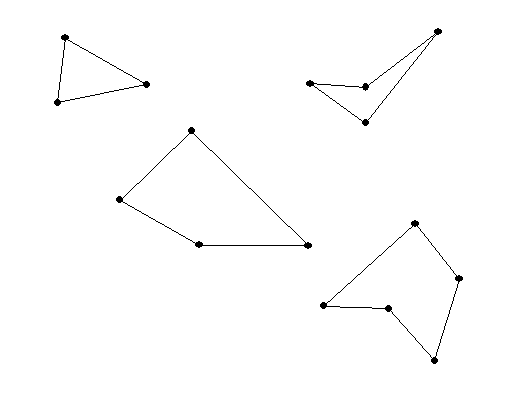
The sum of the measures of the interior angles of a triangle is 180 degrees.
Question:What about a quadrilateral? and a pentagon? or an n sided polygon ( an "n -gon")?
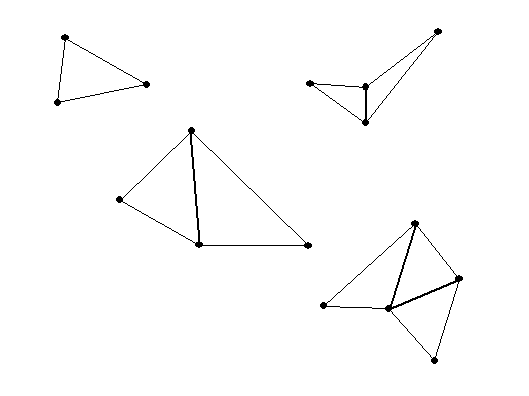
When n = 3 this is a triangle, n=4, a quadrilateral, or
when n= 5, a pentagon.

The sum of the measures of the interior
angles of a triangle is 180 degrees.
Question:What about a quadrilateral? and a pentagon?
or an n sided polygon ( an "n -gon")?

From the figure we saw that for a quadrilateral (n =4), which can be dissected
into two triangles,
the sum is 2*180= 360 degrees.
And for a pentagon (n=5)
which can be dissected into 3 triangles, the sum is 3*180=540 degrees.
A regular polygon is a polygon where the sides are all of equal length and the angles are all congruent (or of equal measure).
For a triangle, the individual angle is __180/3
=_60____ degrees.
For a square, the individual angle is ___360/4=90_____
degrees.
For a regular pentagon.... ____3*180/5 = 540/5
=108__ degrees.
Now for a HEXAGON (6 sides) the sum of the angles
is
____720___ degrees.
So ... for a REGULAR HEXAGON, the individual
angle is 4*180/6 =720/6 =120________ degrees.
| name of polygon | degrees of the interior measure of each angle |
360 degrees divided by # in Column 2 |
|
| equilateral triangle | 60 | 360 / 3 = 120 | |
| square | 4 |
90 | 360/4= 90 |
| regular pentagon | 5 |
3*180/5= 108 |
360/5= 72 |
| regular hexagon | 6 |
4*180/6=120 |
360/6= 60 |
| regular heptagon | 7 |
5*180/7 |
360/7 |
| regular octagon | 8 |
6*180/8=135 |
360/8 = 45 |
| regular dodecagon |
12 |
10*180/12=1800/12=150
|
360/12=30 |
(180 - 360/n) + (180 - 360/k) + (180
- 360/p) = 360
3*180 -360( 1/n+1/k+1/p)= 2*180
1*180 = 360( 1/n+1/k+1/p)
So, for example, n=3, k=4
and p= 5 is not possible since
| Number of polygons around a vertex |
Equation for angle sum = 360 | Equivalent Arithmetic equation | Solutions to the arithmetic equations. | |||||||||||||||||||||||||||||||
| 3: n , k, p | 180 - 360/n+180 - 360/k+180 - 360/p = 360 | 1/n+1/k+1/p =1/2 |
|
|||||||||||||||||||||||||||||||
| 4: n, k, p, z | 180 - 360/n+180 - 360/k+180 - 360/p 180 - 360/z = 360 | 1/n+1/k+1/p +1/z =2/2 =1 |
|
|||||||||||||||||||||||||||||||
| 5: n, k, p, z, w | 180 - 360/n+180 - 360/k+180 - 360/p+180 - 360/z+180 - 360/w = 360 | 1/n+1/k+1/p +1/z+1/w =3/2 |
|