Martin Flashman's Courses - Math 371 Spring, '04
Geometry Notes
[Work in Progress DRAFT VERSION 2-16-04]
Blue sections indicate tentative plans for those dates.
|
Monday
|
Wednesday |
Friday
|
|
|
1/21 Introduction |
1/23 Continue discussion of what is "geometry"?
Start on The Pythagorean Theorem
|
1/26 The Pythagorean Theorem plus...
|
1/28 Dissections
|
|
2/2 M&I's Euclidean Geometry
Begin Constructions and the real number line.
|
2/4 The real number line.
|
2/6Breath
|
|
2/9 Inversion and Orthogonal Circles
|
2/11 Odds and ends, More on Inversion..
|
2/13.Isometries |
2/16 Classification of Isometries & More :)
|
2/18 Finish Classification of Isometries.
|
2/20 Proof of classification result for plane isometries
|
2/23
Symmetry.Begin Similarity & Proportion
|
2/25 More on Proportion and Measurement
|
2/27
Inversion and Beginning to
See The Infinite.
|
3/1 More
on seeing the infinite.
The
Affine Line and Homogeneous Coordinates.
|
3/3 More on The
Affine Line and Homogeneous Coordinates.
|
3/5 More on The
Affine Lineand Homogeneous Coordinates and the Affine Plane
|
3/8 The Affine Plane and
Homogeneous Coordinates
|
3/10 Axioms and Finite geometries!
Connecting Axioms to Models. |
3/12
Connecting Axioms to Models.
|
3/15 No class Spring Break!
|
3/17 No class Spring Break! |
3/19 No class Spring Break!
|
3/22
Introduction
to projective geometry with homogeous coordinates.
Z2 and
Finite Projective Geometry.Video "A non-Euclidean Universe."
|
3/24
Algebraic and Visual Models
for Affine and Projective Geometries.
Introduction to Desargues' Theorem- a result of projective geometry.
|
3/26 Desargues' Theorem- Proof of Desargues' Theorem in the Plane
Axioms for Synthetic Projective Geometry
(see M&I)
|
3/29 More examples of proofs in synthetic projective
geometry.
|
3/31 No class CC Day
|
4/2More examples of proofs in synthetic projective
geometry.
|
4/5 A look at duality and some applications.
.
|
4/7
Conics. Introduction to Pascal's Theorem
Sections and Perspectively related Figures |
4/9 Some key configurations.space
duality, perspective reconsidered.
Video: Orthogonal Projection
|
4/12 Perspectivies as transformations.
The complete Quadralateral.
|
4/14 Quiz 2
Projectivities. Projective relations
Projective Line transformations: Synthetic Projectivities;
|
4/16
Start Isometries
with Homog. Coord.
Matrices
More on Matrix Projective Transformations.
|
4/19 An Inversion Excursion.
|
4/21 Harmonics: uniqueness
and coordinates for Projective Geometry.
Planar transformations and Matrices |
4/23
Quiz #3
Inversion Video.
|
|
4/26
|
4/28 |
4/30
|
|
5/3
|
5/5 |
5/7
|
-
1-21 Introductory Class.
- 1-23
-
Different types of geometry:
-
Euclidean: Lengths are important
-
Similarity: Shape is important
-
Affine: Parallel lines are important.
-
Projective: "Shadows" are important
-
Differential: Curvature is important.
-
Topological: General shape- especially holes and connectedness- is important.
-
What is synthetic geometry? A geometry that focuses on connecting statements
(theorems, constructions) to a foundation of "axioms" by using proofs.
-
What is analytic geometry? A geometry that focuses on connecting statements
(theorems, constructions) to a foundation of number based algebra.
Other aspects of geometry briefly
-
transformations: tools that allow for changing figures:
- Euclidean: translations,
rotations, and reflections.
- Similarity: magnifications, dilations
- Affine: Preserve parallel lines
- Projective: "linear projections"...line preserving
- Differential: "smooth".
- Topology: continuous
-
geometry as an empirical science
-
geometry as a formal system of information
-
geometry focused on special objects like triangles, or special qualities
like convexity.
-
Geometry
on the web and using GSP and Wingeom
2D Introduction.
Another
proof using "shearing" illustrated in the Java sketch below taken from
a Geometers' Sketchpad example can be connected to Euclid's proof..
(Based on Euclid's Proof) D. Bennett 10.9.9
-
Shear the squares on the legs by dragging point P, then point Q, to the
line. Shearing does not affect a polygon's area.
-
Shear the square on the hypotenuse by dragging point R to fill the right
angle.
-
The resulting shapes are congruent.
-
Therefore, the sum of the squares on the sides equals the square on the
hypotenuse.
In considering the Pythagorean theorem, what kind of assumptions were
needed in the first proof with the triangles and squares?
Here are some considerations related to those assumptions:
-
How could we justify identifying "equal" objects (congruent figures)?
-
How do the objects fit together?
-
How do movements effect the shapes of objects.
[Side Trip] Moving line segments:
1-26
-
Consider Euclid's Proposition
1 and Proposition
2.
-
These propositions demonstrate that Euclid did not treat moving a line
segment as an essential property worthy of being at the foundations as
an axiom. However, this is a fundamental tool for all of geometry.
-
Note that in the proofs of these propositions certain points of intersection
of circles are presumed to exist without reference to any of the postulates.
These presumptions were left implicit for hundreds of years, but were cleared
up in the 19th century when careful attention was given again to the axioms
as a whole system.
Notes on Existence: Existence is an often
overlooked quality in a mathematical statement:
For example it is common to state that sqrt(2)
is an irrational number.
The proof starts by assuming sqrt(2) is a rational
number, say sqrt(2) = p/q where p and q are natural numbers, so that
... 2 q2 = p2. From this the usual proof of
the statement deduces a contradiction. However, the statement assumes
that there is a number the square of which is equal to 2.
One can justify the existence of the square root
of 2 in many ways. One way presumes that any line segment has a length
measured by a real number. Then by the pythagorean theorem, the diagonal
of a unit square will have length sqrt(2).
An example of a geometry where circles do not
intersect is given by using the rational coordinate plane.
Points correspond to ordered pairs of rational numbers.
The circle with center (0,0) and radius 2 and
the circle with center (2,0) and radius 2 meet in the ordinary plane at
the points with coordinates (1, sqrt(3)) and (1, -sqrt(3)) . Since sqrt(3)
is not a rational number, this ordered pair does not correspond to a point
in the rational coordinate plane, so the two circles do not have a point
of intersection in the rational coordinate plane.
Another example of a point not in the rational
coordinate plane is the point (sqrt(2),0). This point can be constructed
in the ordinary plane with straight edge and compass using the circle with
center (0,0) and radius determined by the points (0,0) and (1,1). This
circle will meet the X-coordinate axis at the point (sqrt(2),0)
1-28
First, consider some of the background results which were known to Euclid:
(1) parallelograms results and (2) triangle results. The justifications
for these results can be reviewed briefly.
(1) a. Parallelograms between a pair of parallel lines and on the same
line segment are equal (in the sense of being able to decompose one to
reconstruct the other). Proposition
35.
b. Parallelograms between a pair of parallel lines and on congruent
segments are equal (in the sense of being able to decompose one to reconstruct
the other). Proposition
36.
(2) a. The line segment connecting the midpoints of two sides
of a triangle is parallel to the third side and is congruent to one half
of the third side.
[The justification of this result is left
as an exercise in traditional Euclidean Geometry.]
b. By rotating the small triangle created by connecting the midpoints
of two sides of a triangle 180 degrees about one of the midpoints, we obtain
a parallelogram. (This shows that the triangle's area is the area
of this parallelogram which can be computed by using the length of the
base of the triangle and 1/2 of its altitude- which is the altitude of
the parallelogram.)
Compare this with Euclid Prop. 42 and Prop. 44.
1/30
(ii) The triangulation of any polygonal region
in the plane is a key element in a proof of the equidecomposable
polygon theorem.
The proof of this proposition examines a more careful characterization
of the polygonal regions being considered. The key idea of the proof goes
by induction on the number n = the number vertices = the number of sides
in the polygon, as follows:
When n = 3 the result is trivial.
Suppose n> 3 and that for any polygon with k vertices/ sides, where
k<n, the polygon can be triangulated.
Now proceed to consider the vertices, v1, v2, ..., vn ordered
so that vi is adjacent to vi+1 and vn is adjacent to v1.
Take a ray from v1 and rotate it from v1v2 so that it intersects
the inside of the polygon. continue to rotate until it meets another vertex.
Case 1. This vertex is v3. Then consider the polygonal region Q1
= v1v3...vn which has n-1 vertices. By induction Q1 can be triangulated,
so the original polygon is triangulated using the triangulation of Q1 and
the triangle v1v2v3.
Case 2. The vertex is vn-1. Then consider the polygonal region Q2
= v1v2v3...vn-1 which has n-1 vertices. By induction Q2 can be triangulated,
so the original polygon is triangulated using the triangulation of Q2 and
the triangle v1vnvn-1.
Case 3. The vertex is vk with k different from 3 or n. Then consider
the polygonal regions Q3 = v1v2...vk which has k vertices (k<n) and
Q4 = v1vkvk+1...vn which has n-(k-2)<n vertices. By induction Q3 and
Q4 can be triangulated, so the original polygon is triangulated using the
triangulations of Q3 and Q4.
For more discussion of proofs of this proposition see Triangulations
and arrangements, Two lectures by Godfried Toussaint, transcribed by
Laura Anderson and Peter Yamamoto.
How to "add two parallelograms to form a single parallelogram which
is scissors congruent to the two separate parallelograms":
Intersect two pairs of parallel lines, l and l' with m and m'- one
from each of the given parallelograms. Draw a diagonal HI in the resulting
parallelogram.
Cut and translate one parallelogram so that it is scissors congruent
to a parallelogram HIJK within the same parallel lines l and l' with one
side being the diagonal.
Cut and translate the other parallelogram so that it is scissors congruent
to a parallelogram HINO within the same parallel linesm and m' with one
side being the diagonal and on the other side of the diagonal HI from the
transformed first parralleogram. Now draw the parallel NO to the diagonal
in the second transformed parallelogram HINO so that it intersects the
parallels l and l' from the first parrallelogram at the points Pand
Q. This makes one larger parallelogram JKPQ which is scissors congruent
to the original two parallelograms.
Compare this with Euclid Proposition 45.
Follow this link for a proof of the equidecomposable
polygon theorem. or here is a slightly different approach.
The film Equidecomposable Polygons also proves the result:
If two polygonal regions in the plane have the same area, then there
is a decomposition of each into polygons so that these smaller polygons
can be moved individually between the two polygons by translations or half
turns (rotations by 180 degrees).
- 2-2
-
Read the definitions in M&I
section 1.1
REVIEW of basic plane geometry
concepts and definitions based on M&I.
M&I build their foundations for Euclidean geometry on a one to
one correspondence between points on a line and real numbers and the ability
to match angles with numbers between 0 and 180.
Review materials defining rays, segments, angles, triangles,
and planes - started last class in response to Homework related to Interior points.
Review eight of the basic Euclidean constructions
described in M&I
section 1.2. Note that several of these constructions
rely on some foundations that assert the existence of points of intersection
of circles.
Some comments about Constructions: It is important
to notice that constructions also require a justification (proof) that
the construction has in fact been achieved. In proving the constructions
we use some basic euclidean results, such as the congruence of all corresponding
sides in two triangles is sufficient to imply the triangles are congruent
(SSS). [Other basic Euclidean results are SAS and ASA congruence conditions,
as well as the result that corresponding parts of congruent triangles are
congruent (CPCTC).]
It should also be noted that the three transformations
(translation, rotation, and reflection) commonly used in geometry are commected
to constructions as well. For example, to translate a figure by a vector
it would be useful to know how to construct parrallelograms.
[The
midpoint proposition for triangles assigned for homework.]
Note on midpoints: With the construction of
midpoints in Euclidean Geometry, we can show that a Euclidean line segment
has an infinite (not finite) number of distinct points. Furthermore, if
we think of approximating real number distance with points on a segment
after establishing a unit length, then we can construct the position of
a euclidean point as close as we want to the position where a real number
might correspond to a point in that position.
Tangents to circles.
In considering constructions of tangents to circles we use the characterization
of a tangent line as making a right angle with a radius drawn at the point
it has in common with the circle. ( Book
III Prop. 16.) In our construction, not Euclid's (Book
III Prop. 17), we also use the result that any angle inscribed in a
semi-circle is a right angle. ( Book
III Prop. 31.)
Note on Three
Historical Problems of Constructions.
(1) Trisection of an angle: Since it possible
to bisect and trisect any line segment and bisect any angle, the issue
is, is it possible to trisect any angle?
(2) Duplication of a cube: Since it is possible
to construct a square with twice the area of a given square, the issue
is, is it possible to construct a cube with twice the volume of a given
cube?
(3) Squaring a circle: Since it is possible
to construct a square the same area as any given polygonal region, the
issue is, is it possible to construct a square with the same area as a
given circle.
-
The construction of points that correspond to numbers
on a line.
First construct Pk where k is an integer using circles.
We can construct points with fractions using powers of 2 for the denominator
by bisection.
(However, with only bisection we could not construct a point for 1/3
although we could get very close to that point using a binary representation
of that common fraction.)
[Review the construction of a line though a given point parallel to
a given line. See Euclid
I.31.]
We can also construct Pk when k is a rational number using the theory
of similar triangles.
For example: We can bisect or trisect a line segment, giving us the
ability to find points representing rational numbers with denominators
involvingpowers or 2 and 3, such as 5/6, 7/18, etc.
The figure below gives two ways to achieve these constructions. One
can see how to generalize these to allow one to construct points to represent
any rational number on the line so that the arithmetic of numbers is consistent
with the arithmetic of geometry. [Adding segments and adding numbers, etc.]
Consider M&I's constructions of the same correspondence of
integer and rational points. These also rely on the ability to construct
parallel lines.
The continuity axiom for a euclidean line:
-
Any nonempty family of nested segments
will have at least one point in the intersection of the family.
This axiom allows us to make a 1 to 1 correspondence between any real number
and a point on a euclidean line once points have been determined to correspond
to 0 and 1.
I.e., given P0 and P1 for any
real number x there is a point Px where a<x<b
if
and only if Px is between Pa
and Pb and the point Pk . corresponds
to the number k for any rational number k.
The continuity axiom leads to the important result due to G.
Cantor that any
list (possibly infinite) of points in a given segment of a euclidean line
will not have every point in that segment on the list. [Note
that we can make a list of points corresponding to the rational numbers
once a unit length had been established. 1/1,1/2,2/1,1/3,2/2,3/1,1/4,2/3,3/2,4/1,
....]
The continuity axiom can also be used to prove:
If a line, l, (or circle, O'A') has at least one point inside a given circle
OA and one point outside the same given circle then there is of a point
on the line (circle) that is also on the given circle.
Proof outline for the line-circle:Use
bisection between the points on the line l outside and inside the circle
OA to determine a sequence on nested segments with decreasing length approaching
0. The point common to all these segments can be shown to lie on the circle
OA.
Proof outline for the circle-circle:
Draw the chord between the inside and outside points on the circle O'A'.
Use bisection on this chord to determine rays that by the previous result
will meet the circle O'A'. The bisections can continue to determine a sequence
of nested segments with decreasing length approaching 0 and with endpoints
determining one outside point and one inside on O'A' . The point common
to all the endpoints on the chord will determine a point on O'A'
that can be shown to also lie on OA.
Note: The circle-circle result fills in a hole
in the proof of Proposition 1 in Book I of Euclid.
2-9
We spent the class introducing Orthogonal Circles and The inverse of a
point with respect to a circle as well discussing the concept of
convexity of a geometric figure.
-
Convexity: Another brief side trip into the world of convex
figures.
Recall the Definition: A figure F is convex if whenever A and
B are points in F, the line segment AB is a subset of F.The half plane example:Consider the half plane determined by
a line l and a point P not on the line. This can be defined as the
set of points Q in the plane where the line segment PQ does not meet the
line l. Discuss informally why the half plane is convex.
Review the problems on convex figures in Problem Set 1.
Other convex examples: Apply the intersection property [The intersection
of convex sets is convex.] to show that the interior of a triangle is convex.
Show that the region in the plane where (x,y) has y>x2
is convex using the tangent lines to the parabola y=x2 and the
focus of the parabola to determine a family of half planes whose intersection
would be the described region.
Doing arithmetic with constructions in geometry.
Note
that the construction above allows one to construct a point Px' from a
point Px as long as x is not 0 so that x' x = 1.[ Use the circle of radius
1 with center at P0 to construct the inverse point for Px.]
-
The relation of the inversion
transformation with respect to a circle and orthogonal circles.
2-11
Odds and ends...and isometries !
(1) The continuity axiom can also be used to prove:
If a line, l, (or circle, O'A') has at least one point inside a given circle
OA and one point outside the same given circle then there is of a point
on the line (circle) that is also on the given circle.
Proof outline for the line-circle:Use
bisection between the points on the line l outside and inside the circle
OA to determine a sequence on nested segments with decreasing length approaching
0. The point common to all these segments can be shown to lie on the circle
OA.
Proof outline for the circle-circle:
Draw the chord between the inside and outside points on the circle O'A'.
Use bisection on this chord to determine rays that by the previous result
will meet the circle O'A'. The bisections can continue to determine a sequence
of nested segments with decreasing length approaching 0 and with endpoints
determining one outside point and one inside on O'A' . The point common
to all the endpoints on the chord will determine a point on O'A'
that can be shown to also lie on OA.
Note: The circle-circle result fills in a hole
in the proof of Proposition 1 in Book I of Euclid.
(2)
Proposition:If
C2 is orthogonal to C1 (with center O) and A is a point on
C2 then the ray OA will intersect C2 at the point A' where A and A' are
inverses with respect to the circle C1. Click
here for the proof.
We can use this proposition in the following
Constructions: 1.Construct a circle C2 through a given point
B on a circle C1 and a point A inside the circle so that C2 is orthogonal
to C1.
Solution: First construct the inverse A' of A with respect
to C1 and then the tangent to C1 at B and the perpendicular bisector of
AA' will meet at the center of the desired circle.
2. Construct a circle C2 through two points A and B inside
a circle C1 so that C2 is orthogonal to C1.
Solution: This solution is demonstrated in the sketch below.
Isometries:
Definition: An isometry on a line l /plane
π /space S is a function (transformation), T, with the property that
for any points P and Q, d(T(P),T(Q))=d(P,Q) or m(PQ)=m(P'Q').
Now consider Euclid's treatment of the side-angle-side congruence [Proposition
4] and how it relates to transformations of the plane that preserve
lengths and angles.
Such a transformation T: plane -> plane, has T(P)=P',
T(Q)=Q' and T(R)=R' with d(P,Q) = d(P',Q') [distance between points are
preserved] or m(PQ)=m(P'Q') [measures of line segments are invariant].
Review briefly the outline of Euclid's argument for Proposition
4.
Notes:
-
The Side-side-side (SSS) congruence of triangles
(If Corresponding sides
of two triangles are congruent, then the triangles are congruent)
This allows
one to conclude that any isometry also transforms an angle to a congruent
angle.
-
The key connection between the congruence of figures in the plane and isometries:
Proposition: Figures
F and G are congruent if and only if there is an isometry of the plane
T so that T(F) = {P' in the plane where P'= T(P) for some P in F} = G.
You can read more about isometries by checking out this web site:
Introduction
to Isometries.
Some General Features of Isometries:
Proposition: If T is an isometry, then T
is 1:1 and onto as a function.
Proof: 1:1. Suppose that T(P)=T(Q). Then d( T(P),T(Q))=0=d(P,Q)
so P=Q.
onto. Suppose R is in the plane. Consider A,B, and C in the
plane where C is not on the line AB. Then the points T(A),T(B), and T(C)
form a triangle and using the distances d(T(A),R), d(T(B),R), and d(T(C),R),
we can determine a unique point X in the plane where d(A,X)=d(T(A),R),
d(B,X) = d(T(B),R), and d(C,X)=d(T(C),R), so T(X) = R.
-
What information determines an isometry?
Proposition: For an isometry T where T(P)
= P', when we know T(A), T(B), and T(C) for A,B, and C three noncolinear
point , then T(P) is completely determined by the positions of A', B',
and C'.
Proof: In fact we saw that T(B)=B' must be on the circle with center
A' and radius= m(AB), and T(C)= C' must be on the intersection of
the circles one with center at A' and radius = m(AC) and the other with
center at B' and radius=m(BC). Once these points are determined, then for
any point P, P' must be on the intersection of 3 circles, centered at A',
B', and C' with radii = to m(AP), m(BP), and m(CP) respectively. These
three circles do in fact share a single common point because the
associated circles with centers at A,B, and C all intersect at P.
Fact: If T and S are isometries then ST is also an isometry
where ST(P) = S(T(P))= S(P') [T(P)=P'].
Proof : Consider d(ST(P),ST(Q)) = d( S(T(P)),S(T(Q)) ) = d(S(P'),S(Q'))
= d(P',Q') = d(P,Q) .
Line Isometries: Consider briefly isometries of a
line.
1) translations and 2) reflections.
How can we visualize them?
- Transformations figures (before and after lines) T: P -> P';
- Correspondence
figures on a single line;
- Graph of transformation.
Coordinate function. x -> x' = f (x)
Examples: P x -> Px+5 a translation;
P x -> P-x a reflection.
Can we classify them? Is every line isometry either a translation or
a reflection? Why?
Prop.: These are the only isometries of the line.
Proof: Discussed in class. Given A and A', there are only two choices
for B '. One forces the isometry to be a translation, the other forces
the isometry to be a reflection.
2-16
More on line isometries and coordinates:
Use T to denote both the geometric
transformation and the corresponding function transforming the
coordinates of the points. So ... T(x) = x + 5 for the translation example and T(x) = -x for the reflection example.
More generally, a translation Ta :P x -> Px+a would have Ta(x) = x + a and Reflection about the origin can be denoted R0 , R0(x) = -x . What about a general reflection about the point with coordinate c? Rc(x) =? Use a translation by -c, then reflect about 0, and translate back to c. So Rc(x) = Tc(R0(T-c(x))) = Tc(R0((x -c))=Tc(-x+c)= -x + 2c.
Plane Isometries: Consider isometries of a plane.
1) translations 2) rotations and 3) reflections.
How can we visualize them?
- Transformations figures (before and after planes); [Winplot?]
- Correspondence figures
on a single plane; [Wingeometry]
- Graph? [Visualizing 4 dimensions! ]
Coordinate functions?
Remark: An isometry of the plane is completely determined by
the correspondence of three non-colinear points.
The classification
of isometries.
There are at least four types of isometries of the plane: translation,
rotation, reflection and glide reflection. [In fact , we will show that any planar isometry is one of these four types.]
-
What about coordinates and plane isometries?
Coordinates: (a la M&I
I.3) Use any two non-parallel lines in the plane with coincident 0.
Then you can determine "coordinates" for any pont by using parallelograms.[As
indicated previously, all rational coordinates can be constructed from establishing
a unit. This is outlined more thoroughly in the reading in I.3.]
Isometry examples with coordinates in the plane: (See M&I
I.5 and I.6)
Translation: T: P (x,y) -> P(x+5, y+2)
is a translation of the plane by the vector <5,2>. If we use the coordinates
for the point and T(x,y) = (x',y') then x' = x+5 and y' = y+2. We
can express this with vectors <x',y'> = <x,y> + <5,2>. So translation
corresponds algebraically to the addition of a constant vector.
Reflections: Across X-axis RX(x,y) = (x,-y); Across Y axis RY(x,y)
= (-x,y); Across Y=X , R(x,y)=(y,x). Notice that these can be accomplished
using a matrix operation. Writing the vectors as row vectors
|
Matrix for RX
|
Matrix for RY
|
Matrix for R
|
Or writing the vectors as column vectors
| [ |
1 |
0
|
] |
[ |
x |
] |
= |
[ |
x |
]
|
| 0 |
-1 |
y
|
-y |
Matrix for RX
|
and
| [ |
-1 |
0
|
] |
[ |
x |
] |
= |
[ |
-x |
]
|
| 0 |
1 |
y
|
y |
Matrix for RY
|
and
| [ |
0
|
1
|
] |
[ |
x |
] |
= |
[ |
y
|
]
|
| 1 |
0
|
y
|
x
|
Matrix for R
|
Rotations:
If R90 is rotation about (0,0) by 90 degrees, then R90(x,y) = (-y,x).
Question: What is rotation about (0,0) by t degrees?
Hint: What does the rotation do to the points (1,0) and (0,1)? Is this
rotation a "linear transformation?"
More general Question: What about reflection R(A,B) about the line AX+BY
= 0?
|
| [ |
0
|
-1
|
] |
[ |
x |
] |
= |
[ |
-y
|
]
|
| 1 |
0
|
y
|
x
|
| Matrix for R90 |
|
|
| [ |
a
|
b
|
] |
[ |
x |
] |
= |
[ |
ax+by
|
]
|
c
|
d
|
y
|
cx+dy
|
Matrix for rotation by t degrees?
|
Hint:
Consider that P(1,0) will be transformed to
P'(cos(t), sin(t))= (a,c)
and Q(0,1) will be transformed to
Q'(-sin(t), cos(t))=(b,d) |
|
| [ |
a
|
b
|
] |
[ |
x |
] |
= |
[ |
ax+by
|
]
|
c
|
d
|
y
|
cx+dy
|
| Matrix for R(A,B)?
|
|
Proposition: Any plane
isometry is either a reflection or the product of two or three
reflections.
Proof:
Click here!
2-18
Rotations and reflections [review +]:
Question: What is rotation about (0,0) by t degrees?
Hint: What does the rotation do to the points (1,0) and (0,1)? Is this
rotation a "linear transformation?"
More general Question: What about reflection R(A,B) about the line AX+BY
= 0?
| [ |
a
|
b
|
] |
[ |
x |
] |
= |
[ |
ax+by
|
]
|
c
|
d
|
y
|
cx+dy
|
Matrix for rotation by t degrees?
|
Hint:
Consider that P(1,0) will be transformed to
P'(cos(t), sin(t))= (a,c)
and Q(0,1) will be transformed to
Q'(-sin(t), cos(t))=(b,d) |
|
| [ |
a
|
b
|
] |
[ |
x |
] |
= |
[ |
ax+by
|
]
|
c
|
d
|
y
|
cx+dy
|
| Matrix for R(A,B)?
|
Hint: Rotate, reflect, and rotate back!
Composition of Isometries corresponds to Matrix multiplication!
|
Fill in here more on the discussion of finding the matrices that correspond to rotations and reflections:
A key idea is do the work at the origin and then transfer the work elsewhere using "conjugacy": T at a general point or about a general line can be investivated by first translating the problem to the origin, S, performing the related transformation at the origin,T', and then translating the result back to the original position, S-1. That is using conjugacy:
T = S-1 T' S where T' is the relevant transformation
at the origin. This works for rotation and reflections because
the composition of these transformations corresponds to matrix
multiplication.
2-20
Proposition:
(i) The product of two
reflections that have the lines of reflection intersect at a point O is
a rotation with center O through an angle twice the size of the angle between
the two lines of reflection.
(ii) The product of two reflections that have parallel lines of
reflection is a translation in the direction perpendicular to the two lines
and by a length twice the distance between the two lines of reflection.
(iii)The product of three reflections is either a reflection or
a glide reflection.
Proof: We can do this geometrically or using analytic geometry and the matrices!
Watch the video Isometries (Video
# 2576 in Library) .
- Note: The four types of isometries can be characterized completely by the
properties of orientation preservation/reversal and the existence of fixed
points. This is represented in the following table:
| Orientation
Preserving | Orientation
Reversing |
| Fixed points | Rotations | Reflections |
| No Fixed points | Translations | Glide reflections |
Note: The plane isometries form a group: A set together with
an operation (the product or composition) that is
(0) Closed under the operation - the product of two isometries is
an isometry;
(1) The operation is associative - R(ST) = (RS)T or for any
point P, (R(ST))(P) = R( ST(P))= R(S(T(P))) = (RS)(T(P)) = ((RS)T)(P).
(2) The identity transformation (I) is an isometry - I(P)=P.
(3) For any isometry T there is an (inverse) isometry S so TS =
ST = I.
The operation of composition is not necessarily commutative in the
sense that ST is not always the same transformation as TS:
For example
If R1 and R2 are reflections in intersecting lines l1 and l2 then the isometry R1R2 is a rotation about the point of intersection in the opposite direction
to R2R1.
2-23
Old business:The segment connecting midpoints of the sides of a triangle proposition.
An Applications of Reflection:
(i) Here is a problem encountered frequently in
first semester of a calculus course.
The
Carom Problem Can you see how the solution is related to a "carom"
(angle of incidence=angle of reflection)?
(ii) Here is a similar problem about triangles
that is also related to reflections.
Fano's
Problem Can you find the relationship?
Symmetry of a figure: S is a symmetry
of a plane figure F if
S is an isometry with S(F)=F.
Given
a figure F, the symmetries of F
form a subgroup of all the plane isometries, denoted Sym(F).
Example: Consider the figure F=
an given equilateral triangle. We looked at the six symmetries of this
figure and the table indicating the multiplication for these six isometries.
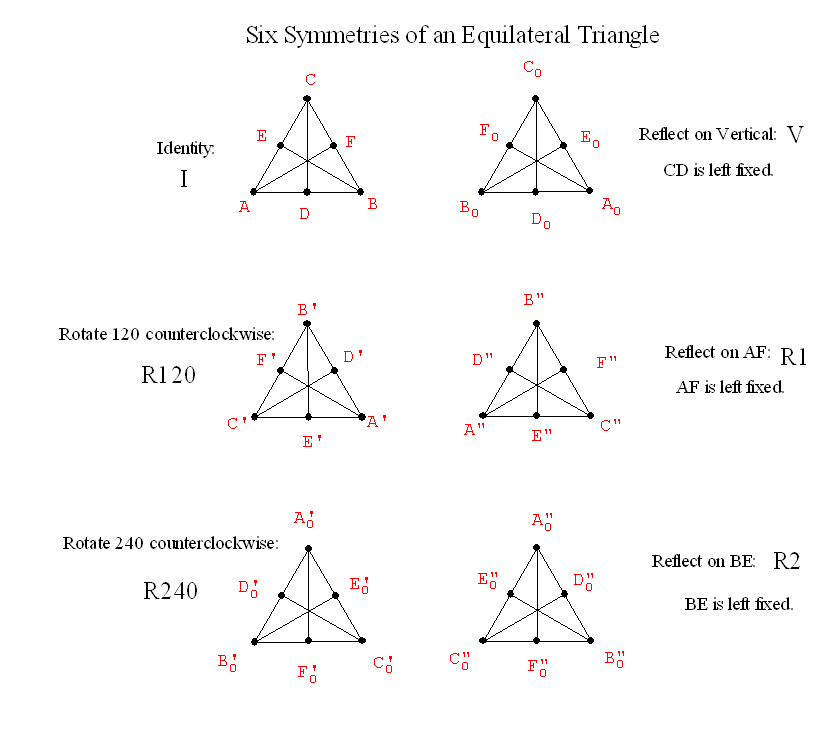
The Group Table of Symmetries of an Equilateral Triangle.
|
R*C
|
I
|
R120
|
R240
|
V
|
R1
|
R2
|
|
I
|
I
|
R120
|
R240
|
V
|
R1
|
R2
|
|
R120
|
R120
|
R240
|
I
|
R2
|
V
|
R1
|
|
R240
|
R240
|
I
|
R120
|
R1
|
R2
|
V
|
|
V
|
V
|
R1
|
R2
|
I
|
R120
|
R240
|
|
R1
|
R1
|
R2
|
V
|
R240
|
I
| R120 |
|
R2
|
R2
|
V
|
R1
|
R120
|
R240
|
I
|
Two interesting sites for looking at symmetries are the Symmetry
Web page and the Symmetry,
Crystals and Polyhedra page.
-
Discussion: What about isometries in three dimensions?
These are generated by spatial reflections in a plane.
A spatial isometry is determined by the transformation of 4 points
not all in the same plane (which determine the simplest 3 dimensional figure-
a tetrahdron!)
Any isometry of space can be expressed as the product of at most 4
reflections.
These results are proven in the same fashion as the comparable results
were proven in the plane.
- Proportions
and similarity:
A look at problems caused by
the diagonal of a square and the issue of finding a unit that would measure
both the side and the diagonal.
First look at the Euclidean algorithm for finding a common segment
with which to measure two segments.
Euclid's original treatment of the "division
algorithm" :
If n and d>0 are integers
then there are integers q and r with
r=0 or 0<r<d where n = q*d +r.
Using q*OD to represent a segment that is made of q segments all congruent
to OD,
Euclid's division algorithm is stated in geometry that of ON is
a segement and OD is a segment that is contained as a subsegment of ON,
then ON is congruent to q*OD with possibly a remaining segment
RN which is congruent to a subsegment of OD.
Repeated use of this algorithm suggests the
Euclidean algorithm for finding a common unit to measure both n and d,
or ON and OD.
If r1=0 or R1N is a point, then OD will be a common unit.
If not apply
the division algorithm to d or OD and r1 or R1N.
If this works to give
r2= 0 or R2N is a point, then R1N will be the common unit.
If not
apply the division algorithm to r1 or R1N and r2 or R2N.
If this works
to give r3= 0 or R3N is a point, then R2N will be the common unit.
If not
continue.
In common arithmetic since each remainder that is not zero is
smaller than the previous remainder, eventually the remainder must be 0
and the process will end- finding a common divisor of the original d and
n.
In the application of the euclidean algorithm to the diagonal and side
of a square, the procedure appears to stop.
However, we can show that because of the fundamental theorem of arithmetic,
it would be impossible to find a segment with which to measure both the
side and the diagonal of a square.
The impact of this on geometry was that
one could not presume that all of geometry could be handled by using simple
ratios of whole numbers for measurements.
[A geometry based on ratios alone
would not permit one to accomplish proposition 1 of Book I of Euclid! since
this would mean that the geometry would have to be able to have ratio involving
the sqr(3) - which like the sqr(2) is also an irrational number.]
- 2-25
- A major part of geometry before Descartes was Euclid's
(Eudoxus') resolution of the issue in Book
V def'ns 1-5.(Joyce)
- Definition 1
- A magnitude is a part of a magnitude, the less of the greater, when it measures the greater.
- Definition 2
- The greater is a multiple of the less when it is measured by the less.
- Definition 3
- A ratio is a sort of relation in respect of size between two magnitudes of the same kind.
- Definition 4
- Magnitudes are said to have a ratio to one another which can, when multiplied, exceed one another.
- Definition 5 (Byne's)
- Magnitudes are said to be in the same ratio, the first
to the second and the third to the fourth, when,
if any equimultiples
whatever are taken of the first and third,
and any equimultiples
whatever of the second and fourth,
the former equimultiples alike
exceed, are alike equal to, or alike fall short of, the latter
equimultiples respectively taken in corresponding order.
Look at these definitions and note some key items:
* Ratios exist only between magnitudes of the same type. (This is usually
described as "Homogeneity".)
* For ratios to be equal the magnitudes must be capable of co-measuring.
* Euclid's axioms do not deny the existence of infinitesimals- but
will not discuss equality of ratios that use them.
Briefly: An infinitesimal segment is a segment AB that is so short that it would appear to be coincident points and for any k, k*AB cannot contain
any ordinary segment determined by points that we can see as distinct!
In the history of mathematics, infinitesmal segments we used in the early
developments of the calculus. For example: Consider the ratio of {the change in the area
of a square, A(s), when the length of a side, s, is changed by an infinitesimal,
ds} to {the change in the length of the side, ds}. That is: What is [A(s + ds) - A(s)]/ds ? Answer: [2s*ds + ds*ds]/ds
= 2s +ds. Thus the ratio would be indistinguishable as a number from 2s.
]
* The Axiom of Archimedes that says that for any two segments one can be used to measure the other. [There are no infinitesimals
for an Archimedean geometry.]
Example: Euclid uses the theory of proportion in Book VI, Proposition
1 and Proposition
2.
(see also Byrne's Prop.1 and Prop.2.)
The connection [between Euclid's definition of proportionality
(equal ratios) and real number equality of quotients].
We can show the following
Proposition: For segments A,B,C,and D with m(A)=a,m(B)=b,
m(C)=c, and m(D)=d:
-
If A:B::C:D then a/b=c/d .
-
If a/b=c/d then A:B::C:D
Proof.
-
The concept of similarity as a transformation.
Consider a similarity on a line with a center of similarity and a given
positive magnification factor. This leads to a consideration of the effect
of a similarity on the coordinates of a point on the line.
If we use the point P0 for the center, then we see that the similarity
T with magnification factor of 2 would transform Px to P2x, or T(Px)=P2x.
Removing the P from the notation we have T(x)=2x. Using T(Px)=Px', we find
that T is described by the correspondence where x'=2x.
With the center at another point, say P3, the transformation T* is
controlled by the fact that T*(Px) - P3 = 2(Px-P3).
So that for T* we have x' = 3 + 2(x-3).
We can also observe that if we let S(Px)=P(x-3) and S-1(Px)=P(x+3)
then
S-1(T(S(Px))) = S-1(T(P(x-3)))
= S-1(P2(x-3))
= P(3+2(x-3))
= T*(Px),
so S-1TS=T*.
2-27
Old business again: The segment connecting midpoints of the sides of a triangle proposition: A Vector Proof
Central similarities in the plane:
In the plane, a similarity Tm with factor m and
center
at (0,0) will transform (x,y) to (mx,my). Thus x' = mx,
and y' = my are the equations for this transformation. This transformation
can be represented using a matrix as follows:
| [ |
m
|
0
|
] |
[ |
x |
] |
= |
[ |
mx |
]
|
| 0 |
m
|
y
|
my |
Matrix for Tm
|
Other central similarities T* with factor m and center at (a,b)
can be recognized as related to Tm by using the translation S(x,y)
= (x - a,y - b) and seeing that S-1TmS=T*.
[Try this yourself!]
Proposition: If l is a line in the plane with equation
AX+BY=C with A and B not both 0, then the set l' = {(x',y'):
Tm(x,y)=(x',y') for some (x,y) on l} is a line in the plane that
is parallel to l (unless l = l' ).
Proof (outline): Show that the equation of l' is
AX+BY = mC.
View video (in Library #4376 ) on "Central
similarities" from the Geometry Film Series. (10 minutes)
View video (in Library #209 cass.2) on
similarity (How big is too big? "scale and form") "On Size and Shape"
from the For All Practical Purposes Series. (about 30 minutes)
Mean Proportions in right triangles and Inverses:
Consider a right triangle ABC with hypotenuse AB. Notice that if the altitude
CD is constructed with the hypotenuse AB as the base, the figure that results
has 3 similar right triangles. ABC, ACD, and DCB. Using similarity of these
triangles we see that there is a proportion of the segments of the hypotenuse
AD, DB and the altitude CD given by AD:CD::CD:BD. If we consider the lengths
of these segments respectively as a,h, and b then the numerical
proportion may be expressed as a/h=h/b or using common algebra ab=h2.
Notice this says that h = sqrt(ab).
Application of this construction:
1. Choosing a = 1, this proportion becomes h
= sqrt(b)
2. Choosing h = 1, this proportion becomes a =
1/b
or ab = 1.
-
Note: Since ab=h2, the construction of the segment
with length h solves the problem of constructing the side of a square
which has the same area as a rectangle with sides of length a and
b.
So we can square a rectangle with straight edge and compass tools.
(See
the three historical problems of constructions and the dissection theorem discussed earlier.)
-
The arithmetic mean of two segments of length a and b
is a segment of length m with the property that m - a =
b - m, so that a+b = 2m.
The geometric mean of two segments of length a
and b is a segment of length h with the property that h/a=
b/h or ab=h2-
- This brings up again the concept of inverses of points with respect to a given
circle and the construction of inverses. These are connected to the
similar triangles just mentioned showing that the constructions in M&I
1.3 were correct.
-
Here is one construction of an inverse using the previous facts about similar
right triangles.
And here is the construction as given in Meserve and Izzo with x
> 1.
-
Separate
notes on the Affine line and Plane.
3-1
These notes describe such issues as
3-3 And 3-5
-
Homogenous
coordinates for an affine line or plane (in 1 and 2 dimensions).
-
Line coordinates: The ordinary coordinate
(x) for a point Px corresponds to homogeneous coordinates <x,1>=<a,b>
so x=a/b with b not 0. Homogeneous coordinates for a point on a line are
related to lines through the origin. <a,b>=<d,e> if and only if
there is some k, not 0, where d=ka and e=kb. These coordinates correspond
to a line in the ordinary plane passing through (0,0). Except for the line
Y=0, each of these lines has exactly one point on it of the form
(x,1). A point with homogeneous coordinate <a,0> corresponds
to the ideal (infinite) point on the affine line. A similar treatment works
for planar coordinates.
3- 8
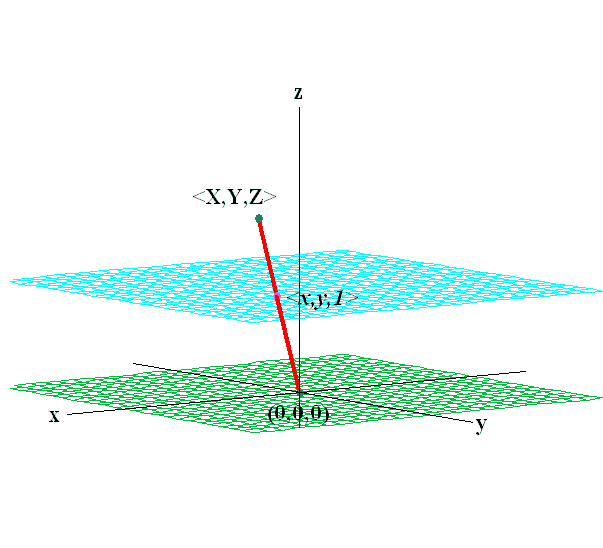
Plane coordinates: The ordinary coordinates (x,y) corresponds to homogeneous
coordinates <x,y,1>=<X,Y,Z> so x=X/Z and y=Y/Z with Z not 0. A point
with homogeneous coordinate <X,Y,0> corresponds to an ideal point in
the affine plane.
A line in the Euclidean plane corresponds to an equation relating the ordinary coordinates of points on the line.
For example, 3x - 5y = 1. To understand this line in
the Affine plane we consider points with homogeneous coordinates <X,Y,
Z> that statisfy the equation: 3X - 5Y = Z or 3X - 5Y - Z = 0.
Notice that using the coordinates <x,y,1> we can
see the relation between the equation for the line in ordinary coordinates
and the equation for the line in homogeneous coordinates. The equation 3X
- 5Y - Z = 0 is described as a homogeneous linear equation.
- Another example of vector methods in geometry: Show that the perpendicular bisectors
of any triangle meet in a single point [which is equidistant from the
vertices][?]
-
3-10
- An introductory discussion of the role of axioms and postulates
in mathematics and the sciences.
In the history of geometry, there have been two main
approaches to the nature of the postulates or axioms for geometry.
1. The postulates are a part of an attempt to model the
reality of measurements on planes and in space. They are a convenience
that allows one to verify only a few limited statements in the modelling
process and then deduce from those postulates other properties of the reality
of the model. Under this view, geometric axioms might be considered similar
to other scientific statements that are supposed to reflect in a platonic
world what we find in the empirical world of reality.
2. Axioms provide linguistic and mathematical structures
useful for developing further logical relations that can be used to understand
a variety of contexts in some abstraction efficiently. An example of such
a view of axioms is found in the axiomatic description of a vector space
and the study of abstract vector spaces, which can be modelled in
the physics view of vectors as things with magnitude and direction, or
in as abstract a setting as the collection of all real valued functions
on a given set S.
-
Here are some examples of "structures" in a variety of settings.
-
A
Geometric structure
-
A triangular graphical geometry.
3-12
Connecting Axioms to models.
3-22
Informal projective geometry and algebra
Projective Geometry with a field with two elements:
Another model for the 7 point geometry.
Z2 =
F2
= {0,1}.
[0,0,1], [0,1,0], [0,1,1], [1,0,0], [1,0,1], [1,1,0],
[1,1,1].
Note that <x,y,z> is a point on [A,B,C] if and only if using the vector dot product: (x,y,z)(A,B,C) = 0
This projective plane satisfies the geometric structure
properties described previously in the "7 point geometry".
This can be verified using the following table where an X indcates the point of the column is an element of the line of the row.
|
Lines\Points
|
<0,0,1>
|
<0,1,0>
|
<0,1,1>
|
<1,0,0>
|
<1,0,1>
|
<1,1,0>
|
<1,1,1>
|
|
[0,0,1]
|
|
X
|
|
X
|
|
X
|
|
|
[0,1,0]
|
X
|
|
|
X
|
X
|
|
|
|
[0,1,1]
|
|
|
X
|
X
|
|
|
X
|
|
[1,0,0]
|
X
|
X
|
X
|
|
|
|
|
|
[1,0,1]
|
|
X
|
|
|
X
|
|
X
|
|
[1,1,0]
|
X
|
|
|
|
|
X
|
X
|
|
[1,1,1]
|
|
|
X
|
|
X
|
X
|
|
-
We can visualize the projective plane using F2
with the 7 points of the unit cube in ordinary 3 dimensional coordinate
geometry.
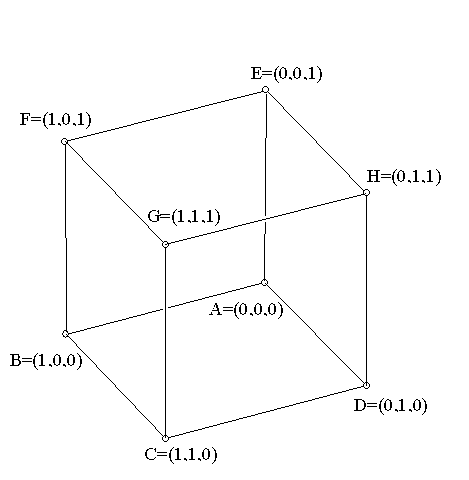
In doing this, many of the lines do correspond to planes
through the origin in the usual geometry, such as [1,0,0] ( X=0) , but
others look a little strange because the use the arithmetic of Z2
, such as [1,1,1] (X+Y+Z=0) which has on it the three point <0,1,1>,
<1,0,1> and <1,1,0> which does not appear to be a plane through the
origin (0,0,0).
-
We watched a video related to axioms for geometry (especially the parallel postulate), The Open University video,
"A non-Euclidean Universe." (#346)
3-24
- Finish watching video.
-
Informal projective geometry and algebra
- Models for geometry using algebra and coordinates for euclidean and affine
geometry especially equations for lines.
- We define a perspective relation:
Two points P and P' are perspectively
related by the center O if O is on the line PP" . Two triangles ABC and
A'B'C' are perspectively related by the center O if O is on the lines AA',
BB', and CC'.
-
3-26
- Another aspect of Projection: Desargues' Theorem in 3-space
and the plane.
Review of the distinctions in our informal discussion of euclidean,
affine and projective geometry.
Euclidean Line: P0 and P1
Euclidean Plane: P(0,0), P(1,0), and P(0,1).
Axes at right angles.
Parallel lines have no point in common. |
Affine Line: P0, P1, and Pinf.
Affine Plane: P(0,0)=<0,0,1>, P(1,1)=<1,1,1>,P(inf,0)=<1,0,0>,
P(0,inf)=<0,1,0>
The Horizon or Ideal line.
Parallel lines meet at an infinite point on the horizon. |
Projective Line: Pinf is treated as an ordinary point. A circle.
Projective plane: All points in the affine plane are treated as ordinary
points. Homogenous coordinates determine all points and lines.
There are no parallel lines. All lines meet. |
In an affine plane constructions using parallel lines in the euclidean
plane can be performed using the horizon line to determine appropriate
parallel lines in the constructions. This was demonstrated by constructing
P(1,0) and P(2,0).
The structure established so far for the affine and projective planes.
In an affine plane there is a special line, the horizon or ideal,
line containing all the ideal infinite points for lines in the ordinary
euclidean plane. Any ordinary line has exactly one ideal infinite point
on it. Two lines in the ordinary plane are parallel if they do not meet.
Two lines in the affine plane are parallel if they meet at the same ideal
infinite point on the ideal line. In the projective plane there are no
parallel lines! We consider these statements in algebraic models for these
planes.
Reconsider how we model geometry visually and
algebraically:
| |
Visual
|
Algebraic
|
|
Euclid
|
 |
Points:Ordinary coordinates(x,y)
Lines: Ax+By+C=0 |
|
Affine
|
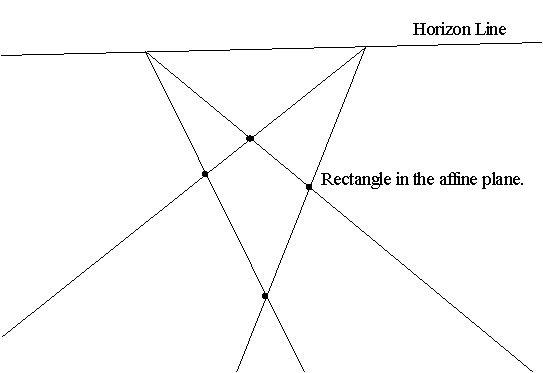 |
Points: Ordinary (x,y) or generally homogeneous <x,y,z> x,y,z not
all 0.
Lines: Ax+By+C=0 or Ax+By+Cz = 0 or [A,B,C] |
|
Projective
|
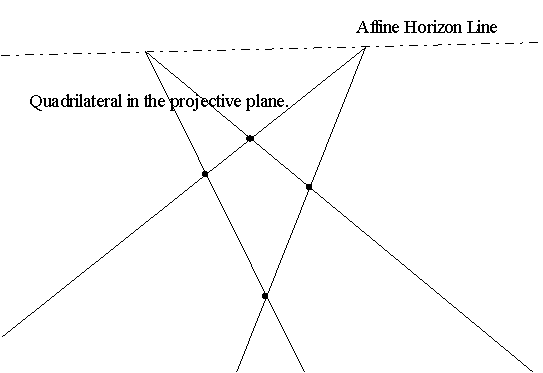 |
Points: <x,y,z> x,y,z not all 0.
Lines: Ax+By+Cz = 0 or [A,B,C] A,B,C not all 0. |
-
The Synthetic Projective Plane can be modelled by the
corresponding visual and algebraic concepts.
3-29
Examples: Two points determine a line:
Visually: Two ordinary points determine an ordinary line. An ordinary
point and an ideal point determine and ordinary line. Two ideal points
determine the horizon line.
Algebraically:
The problem is to determine A, B, and C so that
Ax1+
By1+Cz1=0 and
Ax2+ By2+Cz2=0
- 4/5
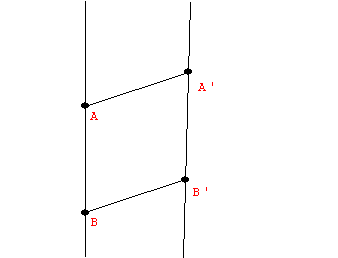
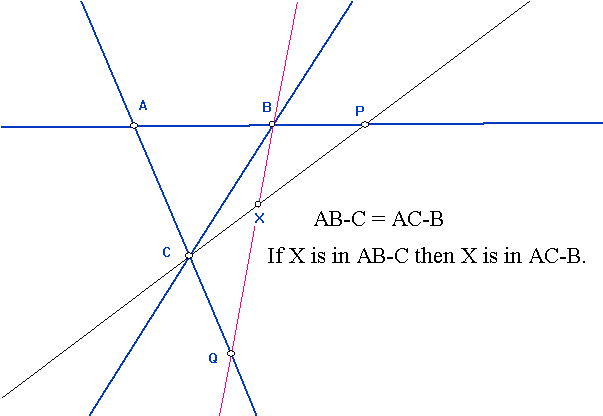
- Proposition: If F and G are points in AB-C then FG is a subset of AB-C
Proof: Suppose X is on the line FG with F and G in AB-C.
Since F is in AB-C,
there is a point P on AB where F is on PC.
Also by work done previously,
we have shown that FG intersects AB at a point Q.
To show that X is in
AB-C, it is enough to show that XC meets AB (at the point R). So consider
the triangle PQF. X is on FQ, C is on PF, so by Axiom 6, XC meets PQ. But
PQ = AB. EOP.
-
If F,G, and Q (not on FG) are in AB-C then AB-C=FG-Q
This is done in two parts. (i) Show that FG-Q is a subset of AB-C.
(ii) Show that C is in FG-Q. [Then by previous work, A and B are also
in FG-Q and by part (i) AB-C is a subset of FG-Q.
Proof:
(i) Suppose X is FG-Q. Then there is a point Z on FG and XQ.

So Z is in AB-C (being on FG) and X is on ZQ (which is in AB-C), so X is in AB-C. Thus FG-Q is a subset of AB-C.
(ii) Left as an exercise. [Hint: Use Postulate 6 to find a point on CQ intersect FG.]
- Proposition: Any two distinct co-planar lines intersect in a unique point. [The proof
of this proposition is an exercise.]
-
Proof
of Desargues' theorem in the projective plane. [Based on the spatial
version of D.T. already proven.]
-
Planar duality and the Converse of
Desargues' theorem in projective geometry.
Duality in Plane Projective geometry:
The axioms for projective geometry in a plane uses two basic objects:
points and lines, and a relation between those: a point is on a line, or
a line passes through a point. The technical term for this relation is
"incident", so we say a point is incident to a line and a line is incident
to a point.
The dual of a statement or description in the context of a projective
plane replaces the word "point" with the the word "line" and the
word "line" with the word "point".
Here are some examples of statements and the corresponding dual statement:
| Two distinct points A
and B are (incident) on a unique lineAB. | Two distinct lines a
and b are (incident) on a unique point
a#b. |
| If the point C is not (incident to) on
the line AB then there are three lines
AB, AC, and BC. | If the line c is not (incident
to) on the point a#b then there
are three points a#b, a#c, and
b#c. |
| The lines AA', BB' and CC' are incident
to the point O. | The points a#a', b#b', and c#c'
are incident to the line o. |
One of the most important logical features of planar projective geometry
is connected to the duality relation. Each of the dual statements for the
postulates for planar projective geometry is a theorem of this geometry.
For example:
Postulate: Given two distinct points there is a line with those points
on it.
Dual Statement and Theorem: Given two distinct lines there is a point
with those lines on it.
As a consequence of this feature, plane projective has a special result
which is about the theorems of geometry and their dual statments.
The Principle of Plane Projective
Duality: Suppose S is a statement of plane projective geometry and S' is
the planar dual statement for S. If S is a theorem of projective geometry,
then S' is also a theorem of plane projective geometry.
The proof of this principle is a proof about proofs.
The idea is that a proof consists of a list of statements about lines
and points.
Each statement in a proof is either one of the postulates,
a previously proven theorem, or a logical consequence of previous statements.
So if we have a proof of a statement S, we have a sequences of statements
A1,A2,...,AN=S.
Each of these statements is either one of the postulates,
a previously proven theorem, or a logical consequence of proevious statements.
Now one can construct the sequence of dual statements A1', A2', ...,
AN' = S'.
With a little argument it can be seen that each of these dual
statements is also either a postulate, a theorem,or a logical consequence
of previous statements.
Here is an application of the principle of duality to Desargues' Theorem.
Desargues' Theorem: (in the (projective) plane). If two coplanar
triangles (determined by points) ABC and A'B'C' are perspectively related
by the center O, then the points of intersection X=(AB)#(A'B'); Y=(AC)#(A'C')
; and Z=(BC)#(B'C') all lie on the same line.
Since Desargue's Theorem uses the hypothesis of a perspective relation
between two triangles, we first look briefly at the dual concept.
Dual of Desargues' Theorem: . If two coplanar triangles (determined
by lines) abc and a'b'c' are perspectively related by the line o, then
the lines joining the points x=(a#b)*(a'#b')'; y=(a#c)*(a'#c') ; and z=(b#c)*(b'#c')
all pass through the same point.
Note on Duality and the concept of perspective: We called two
point triangles ABC and A'B'C' perspectively related
with respect to a point O, if O is
on the lines AA', BB' and CC'.
We define the dual concept by saying that two line triangles
abc and a'b'c' are perspectively related with
respect to a line o, if o passes through
the points a#a', b#b' and c#c'.
Notice that the Dual of Desargues' Theorem is also the logical converse
of Desargues' Theorem. Thus we can say, "The
converse of Desargues' theorem is true by the duality principle."
-
4-7
- Conic Curves in the plane. An introduction
in euclidean and projective geometries.
-
Watch the video "Conics" [VIDEO #628]. This video outlines two separate
ways to understand the conics as a family of curves using the traditional
"Euclidean" views of the conics starting with a cross section of a cone.
Two ways to characterize the conics were treated in the video -
i....related to 2 foci (ellipse and hyperbola) or 1 focus (parabola),
ii...related to a focus and directrix which was also related to eccentricity.
Using two spheres tangent at two focii (F1 and F2) to the sectioning
plane that also touch the sides of the cone, the ellipse is characterized
by the distance from a point on the ellipse P having the sum of the distances
PF1 + PF2 a constant,
-
Consider a circle in a plane and a single point in space not on that plane
(the vertex or apex). This will determine a cone, made from the lines passing
through vertex and points on the circle.
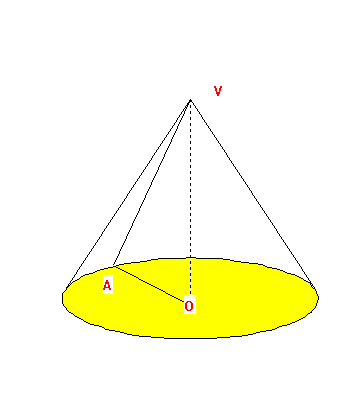
Usually we think of the point lying on the line perpendicular to the
center of the circle and only the part of the cone determined by line segments
from the vertex and the points on the circle. However, the cone does extend
indefinitely beyond the circle and the vertex as the lines extend indefinitely.
When we cut a cone with a plane not passing through the vertex we determine
a curve in the plane, described as the "conic section." [If the plane passes
through the vertex the interesection can be a point (the vertex itself),
a single line element of the cone, or a pair of intersecting lines that
meet at the vertex.] These are circles, ellipses, parabolas, and hyperbolas.
while the hyperbola has the magnitude (absolute value) of the difference
of these lengths |PF1-PF2| a constant. 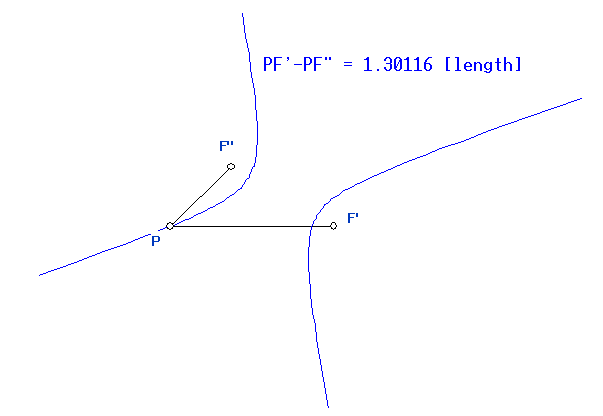
The parabola which in a sense lies between the ellipses and hyperbolae
does not fit into these descriptions using two focii. The film explains
and visualizes these relations.
-
Again using the spheres and choosing one of these, consider the plane through
the sphere at the circle where the sphere touched the cone. This plane
meets the sectioning plane on a line, called the directrix, d. The
film shows that for any conic section a point P on the conic is characterized
by considering the ratio of PF1 to the distance from that point to the
directrix, Pd . This ratio (called the eccentricity of the conic,
e) is a constant for each conic section, in fact the ratio of the sines
of the angle between the sectioning plane and the circle's plane and the
angle between the cone and the circle's plane.
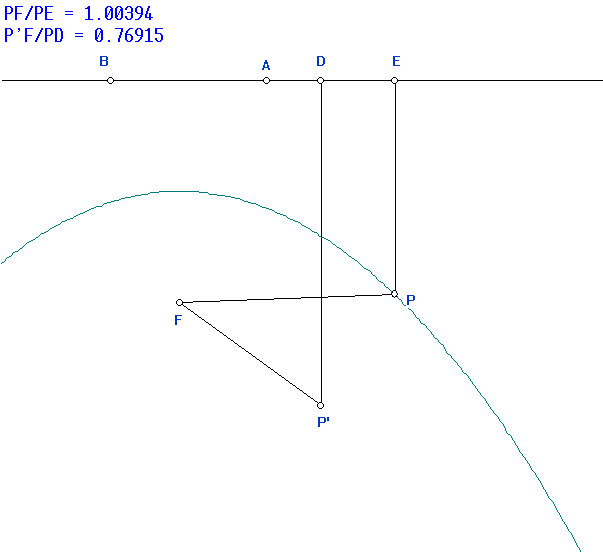
The following table summarizes the results related to this ratio. It
is fully explained in the video.
|
ratio = e
|
Conic
|
|
e = 0
|
circle
|
|
0 < e < 1
|
ellipse
|
|
e = 1
|
parabola
|
|
e > 1
|
hyperbola
|
-
Notice that from the ideas of projection from the vertex of the cone, any
of the conic sections could be the shadow cast by the circle on a sectioning
plane.

- 4-9
- Sections and Perspectively related figures in
a projective plane.
- View Video: Orthogonal Projection [HSU Video #4223] - connect this to central projections and conics.
-
Motivation: In the study of surfaces and solids in 3 dimension (as
in the 3rd semester of calculus) one key method for undertanding a fugure
in space is to consider a planar cross-section. This is a planar figure
determined by the intersection of the spatial figure with a plane. Of course
we have examined just this kind of section in our introduction to the conic
sections!
We can consider this as well as what happens when a 3 dimensional object
passes through Flatland.
Examples: A cube has cross sections that might be a point, a
line segment, a triangle, a square, a rectangle, a quadrilateral,
a pentagon, or a hexagon.
A tretrahedron has a cross section that might be a point, a line
segment, a triangle, a square, a rectangle, or a quadrilateral.
-
In a plane we consider a figure to be made up of points and lines. A
section of a planar figure, F, by a line l, where l is not
in the figure F, is a new figure consisting of the points P where
P = l # m for any m, a line in the figure F.
Examples: The section of the triangle abc by the line
l,
is the set of three points on l , {a# l,b# l, c# l}.
The section by a line l (not passing through the point O) of
a pencil of lines on the point O is the set of points on l with
one point for each line in the pencil.
The dual concept for section: A section of a planar figure, F, by
a point L, where L is not in the figure F, is a new figure
consisting of the lines p where p = L*M for any M,
a point in the figure F.
Examples: The section of the triangle ABC by the point L, is
the set of three lines on L , {A*L,B*L, C*Ll}.
The section by a point L (not lying on a line o) of a
pencil of points on the line o is the set of lines through L with
one line for each point in the pencil.
Point Perspective in the Plane: Figure F is perspectively
related to Figure F' by the point O if there is a correspondence of the
points of F with those of F' so that for any corresponding points, A in
F and A' in F', the point O lies on the line A*A' .
Notes: 1. If the figure F and the figure F' are similar because
of a central similarity, then F and F' are prespectively related.
2. If the figure F and the figure F' are congruent because of a
translation, then F and F' are prespectively related by the point on the
horizon line determined by the translation vector.
3. If the figure F and the figure F' are congruent because of a
rotation by 180 degrees about the center O, then F and F' are prespectively
related by the point O.
Line Perspective in the Plane: Figure F is perspectively related
to Figure F' by the line o if there is a correspondence of the line
of F with those of F' so that for any corresponding lines, m in
F and m' in F', the line o passes through the point m#m'
.
Notes:1. If the corresponding lines in two figures F and F' are
parallel, then in the affine plane, these corresponding lines will meet
on the horizon line. Thus these figures are perspectively related in the
projective plane.
2. If the figure F and the figure F' are congruent because of a
reflection, then F and F' are prespectively related by the line of reflection.
4-12
A look at perspective of planar figures with respect to a center O and
its dual: perspective of planar figures with respect to an axis o.
- Java Figures for 4-12.
- Perspective relation as a transformation: Given O and point ABC on a line
l,
and
a second line l', we can determine unique points A'B'C' on
l'
by A' = OA#l',
B' =OB#l', and C' = OC#l'. Thus A'B'C' are perspectively
related to ABC with center O.
-
-
SKIP THIS FOR NOW:A side trip to planar graphs and dual graphs.
The term duality is used in many different mathematical contexts.In
the study of planar graphs we can find an example of the use of "duality"
that illustrates some of the aspects of mathematical duality.
A planar graph consists of a finite set of points called vertices, line
(straight or curved) segments with these vertices as endpoints called edges,
enclosing planar sets called regions. We can think of these regions as
geographic states, the edges as boundaries between land sections, and the
vertices and places where these boundaries meet.
So a planar graph G is a set of vertices, edges, and resulting regions
in the plane.The dual graph of G is another graph, which for now we'll
denote D(G). D(G) consists of a vertex for each region in G, a region for
each vertex in G, and an edge for each edge. If R is a region in G, we
choose a point in R, call it r, as a vertex of D(G). For each edge, E,
of G, with regions R1 and R1 bordering on E, choose an edge, e, between
r1 and r2 that crosses E. Finally, suppose V is a vertex of G. consider
the edges that end at V and the regions that border these edges. Then these
regions and edges correspond to vertices and edges of D(G) that surround
a region which we'll denote v.
The graph D(G) consists of the vertices, r, edges, e, and regions v
just described.
One aspect of the dual graph is that information about it is revealed
by knowing information about the graph G. For example, if G has 5
regions, the D(G) has 5 vertices.
If G has 7 edges then D(G) also has 7 edges, and if G has 4 vertices,
then D(G) has 4 regions.
The feature that duality exposes here in these statements is the replacement
of the word "vertex" in the statement
about G with the word "region" in the
statement about D(G) and the word "region"
in the statement about the graph G with the word "vertex"
in the statement about D(G).
Duality and Sections in Space.
-
Duality in space: objects are points, lines, and planes.
Consider duality with spatial figures: the platonic polyhedra, tetrahedron,
cube, octahedron,dodecahedron, icosahedron.
Point - plane duality... leaves line-line.
S: A point C and a line AB determine a plane (The join or span AB-C)
.
S': A plane ABC and a line l determine a point (the intersection
ABC#l).
This duality is more like the graph duality discussed earlier.
Notice that as figures, the cube and octahedron are dual, the octahedron
and icosahedron are dual, and the tetrahedron is dual to itself.
Take
a look a this web site on Duality and Polyhedra
Here is a table showing the five platonic solids and the
duality relation of Vertices, Edges (lines), and Faces (Planes):
|
|
V |
F
|
E
|
|
Cube
|
8 |
6
|
12
|
|
Octahedron
|
6 |
8
|
12
|
|
Icosahedron
|
12 |
20
|
30
|
|
Dodecahedron
|
20 |
12
|
30
|
|
Tetrahedron
|
4
|
4
|
6
|
Sections in Space:
Section by a plane: Suppose F is a figure in space consisting
of points, lines, and planes. Suppose p is a
plane that is not containing any element of the figure F. Then the section
of F by p is the figure with lines determined
by the intersection of the planes in F and p
, the points detemined by the intersection of the lines in F with p
.
Example. The figure F is a tretrahedron. p
is a plane that is distinct from the tretrahedron's elements. The section
consists of 4 lines and the six points where those lines intersect in the
plane of p .
Section by a point: Suppose F is a figure in space consisting
of points, lines, and planes. Suppose P is a
point that is not contained in any element of the figure F. Then the section
of F by P is the figure with lines determined
by the join of the points in F and P , the planes detemined
by the join of the lines in F with P .
Example. The figure F is a tetrahedron. P is a point that is
distinct from the tetrahedron's elements. The section consists of 6 planes
and the 4 lines where those lines join the vertices to the point P .
-
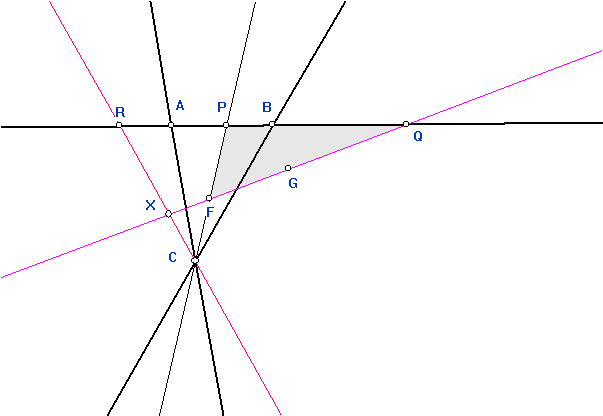
Quadrangles and quadrilaterals in the projective plane.
A Triangle is a figure composed of three points and three lines
in the plane. A triangle is a planar self- dual figure. This is not so
for a quadrangle and quadralateral. We look at the simple quadrangle and
quadralateral, and the complete quadrangle and dual quadralateral, which
has three diagonal points.
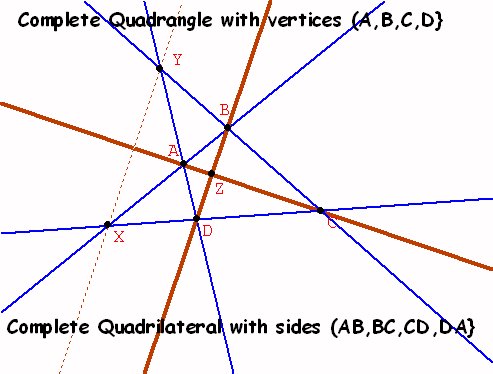 The Complete Quadrangle:
The Complete Quadrangle:
4 points {A,B,C,D} determine
6 lines {AB,AC,AD, BC, BD, CD}
and three additional points
{X,Y,Z}. |

The Complete Quadilateral:
4 lines {AB, BC, CD,AD}determine
6 points {A,B, C, D, X,Y}
with three additional lines{AC,
BD, XY} . |
Postulate: The three diagonal
points in a complete quadrangle do not lie on the same line.
[This eliminates
the 7 point geometry as a projective geometry with these axioms.]
4-14
Duality and graphs in the ordinary plane.
Watch the video on Central Perspectivities.VIDEO4206
A basic principle is discussing graphs of equations and functions in
the plane is the following:
Any point described by coordinates is intersection of two lines, any
line is the join of two points.
Curves in the study of calculus are figures determined by points. For
example the line with equation y= 2x+1 is a figure made up of points with
coordinates of the form (a, 2a+1). Each of these points is determined by
the intersection of two lines: X= a and Y = 2a+1.
We can dualize this figure to a figure made up of lines determined
by the join of points (on two separate axes) x=a and y = 2a+1. Notice
that in the original line figure all the points lie on the same line, while
in the dual figure all the lines will pass through a common point. Thus
this second figure shows a perspective relation between the corresponding
points on the two axes.
We can consider other function relations in this dual visualization
as perspective relations (or perspective functions).
For example f(x) = x + 1 [or f(x) = x] are perspective between the
parrallel axes detemined by the appropriate point at infinity in the affine
(projective) view.
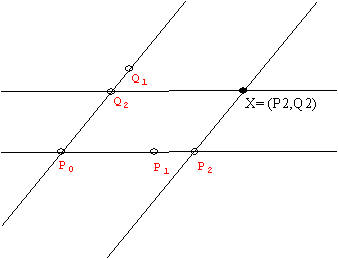
Definition: The product (composition) of two
or more perpectivities is a called a projectivity.
Is we compose two perspectivities we can transform points on a line
to a second line and then back to the original line.
Thus the map from a euclidean, affine, or projective line to itself
can be a projective transformation.
Example: Translation of a euclidean coordinate line by adding
1 unit.
T(Px) = Px+1 can be realized as a product of
perspectivities in the affine plane.
First use the perspectivity that transforms the point Px
on the X axis to the point P' = Qx+1 on the Y axis.
Then use the perpectivity that transforms the point Qx+1
on the Y axis back to Px+1 on the X axis.
Then if T represents the product of these two perspectivities, T(Px)
= Px+1.
Other examples of projective line transformations include central reflection [Use P' =Q -x
for reflection about 0]and central similarities [Use P' =Q ax
for central similarity with scale a and center 0].
4-16
More on projectivities.
The set of projectivities can be considered a group (as did isometries)
, i.e. a set together with an operation which satisfies certain nice algebraic
properties: closure, associativity, an identity and inverses.
Curves are determined by lines.
Projective relation and projectivity between points on lines (and dual)
and then points on a single line.
-
Transformations with homogeneous coordinates of a line. Consider
the isometry T of an ordinary (or affine) line with coordinates that translates
a point 3 units to the right. This isometry is indicated using the
ordinary coordinates by T(Px) = Px+3 or T(x) = x + 3. If we wish to understand
this isometry using the homogeneous coordinates of an ordinary point we
recognize that Px = <x,1>. So for an ordinary point, T(<x,1>)
= <x+3,1>.
Notice that the ideal point will be left fixed by this translation,
that is, T(<1,0>) = < 1,0>. In fact the ideal point is the only fixed
point of translation. We can see this also by looking at the visualization
of the translation construction on an affine line.
But what is the algebra for this isometry when using arbitrary homogeneous
coordinates for an ordinary point?
T(<a,b>) = T(<a/b, 1>) = <a/b + 3,1> = <a+3b,b>.
In summary, T ( <a,b>) = <a+3b,b>.
Notice this formula works as well for the ideal point on the affine
line.
This work can be done using some ideas from linear algebra. Recall
that in matrix multiplication:
| [ |
1 |
3 |
] |
[ |
x |
] |
= |
[ |
x+3 |
] |
| 0 |
1 |
1 |
1 |
and
| [ |
1 |
3 |
] |
[ |
a |
] |
= |
[ |
a+3b |
] |
| 0 |
1 |
b |
b
|
So the isometry of translation can be considered from the point of view
of algebra with homogeneous coordinates:
Treat the coordinates as a column vector, multiply by a square matrix,
and then using the components of the resulting column vector as the homogenous
coordinates for the transformed point.
More on line transformations with homogeneous coordinates .
These transformations can be identified with matrix multiplications
since A(cv)=cAv for A a matrix, c a scalar, and v a column vector so that
pairs of homogeneous coordinates for one point are transformed to homogeneous
coordinates for a single point.
Translation: T(x)=x+3 became
| [ |
1 |
3 |
] |
[ |
a |
] |
= |
[ |
a+3b |
] |
| 0 |
1 |
b |
b
|
Reflection R(x)= -x uses the matrix
Thus
| [ |
-1 |
0 |
] |
[ |
x |
] |
= |
[ |
-x |
] |
| 0 |
1 |
1 |
1 |
and
| [ |
-1 |
0 |
] |
[ |
a |
] |
= |
[ |
-a |
] |
| 0 |
1 |
b |
b
|
Dilation by a factor of 5 M(x)=5x uses the
matrix
Thus
| [ |
5 |
0 |
] |
[ |
x |
] |
= |
[ |
5x |
] |
| 0 |
1 |
1 |
1 |
and
| [ |
5 |
0 |
] |
[ |
a |
] |
= |
[ |
5a |
] |
| 0 |
1 |
b |
b
|
Inversion I(x)=1/x uses the matrix
Thus
| [ |
0 |
1 |
] |
[ |
x |
] |
= |
[ |
1 |
] |
| 1 |
0 |
1 |
x |
and
| [ |
0 |
1 |
] |
[ |
a |
] |
= |
[ |
b |
] |
| 1 |
0 |
b |
a
|
Notice that composition of these matrix transformations corresponds
to matrix multiplication.
4-19
Definition: A general
projective transformation of a projective line is a transformation that
can be expressed using homogeneous coordinates for the points on the line
and an invertible 2x2 square matrix.
If we let the matrix of these transformation be denoted by
Because T is an invertible matrix, the determinant
of T= ad-bc is not zero, and conversely if the determinant
of T= ad-bc is not zero, then the matrix T defines
a projective transformation.
| [ |
a |
b |
] |
[ |
x |
] |
= |
[ |
ax+b |
] |
| c |
d |
1 |
cx+d |
and
| [ |
a |
b |
] |
[ |
x |
] |
= |
[ |
ax+by |
] |
| c |
d |
y |
cx+dy
|
Thus x' = ax+by and y' = cx+dy.
We have shown now that all the transformations of the line we had previously
discussed in the course were examples of this general type of projective
transformation.
Notice that the transformation defined by the matrix T
does
not depend on which of the homogeneous coordinates are used to represent
a point, since
| [ |
a |
b |
] |
[ |
ax |
] |
= |
a[ |
ax+by |
]. |
| c |
d |
ay |
cx+dy
|
Furthermore the matrix aT defines
the same transformation as T for a similar reason since
| a[ |
a |
b |
] |
[ |
x |
] |
= |
a[ |
ax+by |
]. |
| c |
d |
y |
cx+dy
|
Noticing again that ad-bc is not zero, at least
one of the entries, say a, in the matrix is not zero. Using
a
= 1/a we can assume that the matrix has the form
Focus Question: Which transformations leave the
point at infinity fixed?
Analysis: Using homogeneous coordinates for the point at infinity,
it can be expressed as <1,0>.
The question is: For what matrices will this point be transformed
to a point with homogenous coordinates of the form <?, 0> where ? is
not zero?
For this to be true, we can see from the matrix equation
| [ |
1 |
b |
] |
[ |
1 |
] |
= |
[ |
x |
] |
| c |
d |
0 |
0 |
that c = 0. Thus the transformations that leave the point
at infinity fixed are of the form,
Then recognizing that the determinant of T is not 0, it follows
that d is also not 0.
Thus these transformations are of the form on a finite point:
:
| [ |
1 |
b |
] |
[ |
x |
] |
= |
[ |
x/d+b/d |
] |
| 0 |
d |
1 |
1
|
and
| [ |
1 |
b |
] |
[ |
x |
] |
= |
[ |
x+by |
] |
| 0 |
d |
y |
dy
|
Thus this transformation involves a translation if b is not
0, and a similarity of d is not 0.
An overview of what we've considered
so far-
Euclidean Geometry
lines/planes
|
Affine geometry
lines/planes
|
Finite geometry
lines[/Planes?]
|
Projective Geometry
lines/planes
|
Axioms
Euclid
Hilbert |
No Axioms Yet
A figure indicating
an ideal point or line |
Axioms
7 points/7lines
A figure. |
M & I Axioms
A figure indicating
all points and lines |
| Parallel lines don't meet |
Parallel lines meet
at an ideal point. |
All pairs of lines
have a common point |
All pairs of lines
have a common point |
Coordinates
Analytic/Algebraic |
Ordinary coord's
w/ infinite (ideal) points
Homogeneous Coordinates |
Homogeneous Coordinates
with coefficients in {0,1}= Z 2 |
Homogeneous Coordinates
with real number coefficients |
Transformations
Isometries
Similarities |
Similarities |
? |
perspective and projective transformations. |
-
Consider the construction of P1/2 from P0, P1 and P¥
on
the projective line. This begins a discussion of a
harmonic relation
between four points on a line. We observed that in fact the relation
was present between P0, P1, P¥
and P1/2.
Harmonic Relation of 4 points on a line. [This will be
the chief tool used to introduce (homogeneous) coordinates into projective
geometry.]
Four points on a line l are harmonically related if the line
is determined by a pair of points from the intersection of lines in a complete
quadrangle and the intersection of that line with the other two sides of
the complete quandrangle.
[In the figure: The line XZ would determined two other points,
XZ#AD=R
and
XZ#BC=S,
so that the points XRZS are harmonically realted.] This would be denoted
H(XZ,RS).
Four points on a line that are harmonically related:
Using the text notation we can show that if H(AB,CD) then H(BA,CD),
and also conversely if H(BA,CD) then H(AB,CD). This is the meaning
of saying "H(AB,CD) is equivalent to H(BA,CD)". Similarly we can show H(AB,CD)
equiv. to H(AB,DC) and H(BA,DC).
One way to see this is to envision relabeling the original quadrangle
to reverse the order in which the points on the line are organized. |
 |
Notice the relation between the "double points"
in the figure and the "single points".
Proposition: H(RT,SU) is equivalent to
H(SU,RT) (adapted from Meserve & Izzo).
I.e.,
if
H(RT,SU) then H(SU,RT).
Proof: Consider the new quadrangle determined on the
figure by the four points P3, P4, W and V.
[To complete the proof we need only show that WV meets SU at the
point T.]
Notice in the figure that Triangle
WP1P2 is perspectively related by the line SU to triangle VP3P4.
Thus by converse of Desargues' Theorem we have that the triangles are perspectively
related by a point. But this point must be T, so the line WV
passes through the point T, completing the demonstration that H(SU,RT).
-
Recall the construction on the affine line of the
point P1/2 from P0,P1, and P¥.
Notice that the figure used to construct P1/2 showed that H(P0 P1,
P¥ P1/2).
-
Notice that the construction on the affine line of the point P2 from
P0,P1, and P¥ looks
the same.
Notice that the figure used to construct P2 showed that H(P1 P¥,
P0 P2).
In general, following this construction of P2, it is possible
to construct a fourth point, D, on a line given A,B and C already on the
line so that H(AB,CD). We can use the construction of P2 to show how
to construct the point D in general. This will be the key to giving a correspondence
between points on a projective line and the real numbers with ¥
as a single additional "number" added to extend the set of numbers.
One key issue then is:
Is the point constructed from the points A,
B and C uniquely determined by the fact that it is in the harmonic relation
with A, B, and C? That is, if A,B, and C are three points
on a line and D and D* are points where H(AB,CD) and H(AB,CD*), then must
D=D*?
This is the question of the uniqueness of the point D. We can prove
that in fact the point D is uniquely determined.
The
proof follows the argument of Meserve and Izzo.
It used Desargues' theorem several times.
There was a discussion of the dual concept of a harmonic relation between
four lines passing through a single point.
Theorem: If A,B,C, and D are on a line l with H(A,B,C,D)
and O is a point making a section with these four points, consisting of
the four lines a,b,c and d, then H(a,b,c,d).
Proof: see M&I Theorem 5.4.
Corollary: (By Duality) If a,b,c, and d
are on a point O with H(a,b,c,d) and l is a
line making a section with these four lines, consisting of the four points
A,B,C and D, then H(A,B,C,D).
Application:
We can think of a perspectivity between points ABCD on line l
and A'B'C'D' on the line l' with respect to the point O as
being a section of the points ABCD by the point O followed by a section
by the line l' of the lines a,b,c, and d on the point
O.
Applying the previous theorem and its corollary we see that: If
H(AB,CD) then H(ab,cd) and thus H(A'B',C'D').
Note: This application shows that if four harmonically related points
on a line are perspectively related to four points on a second line, then
the second set of four points is also harmonically related. Furthemore,
this result can be extended easily to points that are projectively related.
Thus the transformations of projectivity in projective geometry
preserves the harmonic relationship between four points.
[This last note is comparable to the fact that in Euclidean geometry,
isometries preserve length, and in affine geometry that similarities preserve
proportions.]
Harmonic Conjugate as a Transformation: Given A,B, and C points
on a projective line, we have shown that there is a unique point D so that
H(AB,CD). D is called the harmonic conjugate of C with respect to AB.
In many ways this gives a transformation of the point on the line to
other points that is similar in its nature to reflections and inversions.
Notice that on a projective (affine) line two point will cut the line into
two disjoint pieces, as does a single point for reflection and the points
PR and P-R for inversion, where the transformation
maps points in one set into the other while leaving the "boundary points"
fixed.
With the existence and uniqueness of the point D established, we can
now consider some examples illustrating how to establish a coordinate
system for a projective line by choosing three distinct points to be P0,
P1, and P¥.
We can construct P2, P-1, P1/2, (in two different ways).
Exercise: Construction P3 and P1/3.
Show that with the choice of three points on a projective line
we can construct points using harmonics to correspond to all real numbers
(as in our informal treatment of the affine line).
4-26
A brief excursion back to the Inversion Transformation.
5-7:
Projective transformations of the plane and computers graphics.
The complex numbers, C, and the geometry
of CP(1).
The ordinary points on this "line" can be visualized as
a plane or all the points of a sphere except one- which corresponds to
the north pole or the point at infinity. [Compare this to the visualization
of RP(1) as a circle.]
Algebraic projective transformations of CP(1).
This means first examine what it means for these to be affine, and then
the geometry of addition and multiplication of complex numbers.
Addition
of a constant complex number corresponds to a translation.
Multiplication
by a constant corresponded to a dilation using the absolute value of the
complex number for the factor and a rotation by the angle the corresponding
vector mad with the positive real axis.
An affine transformation
can preserve the distance between complex numbers if |a|=1 where
a is a complex number, so a can be thought of a point on the unit circle
and multiplication by a corresponds to a rotation. So affine transformations
of CP(1) are rotations followed by translations of the plane of
complex numbers.
What about inversions... ?
-
Review of algebraic projective transformations of the real projective line
RP(1).
RP(1) is characterized using the homogeneous coordinates as a set of
pairs of real numbers <a,b>, not both zero, with <a,b>=<c,d> in
the case there is a nonzero real number t so that c=ta and d=td.
The transformations correspond to 2x2 matrices with non-zero determinant.
These form a group under composition (matrix multiplication) and we have
looked at many examples to see how a matrix transforms points on the projective
line with coordinates to other points on the projective line.
We will see how this transformation is completely determined by the
correspondence of three distinct pairs of points. In some cases the transformation
transforms an ordinary point to an ordinary point, the ideal point to an
ordinary point and an ordinary point to the ideal point, and in some cases
the transformation will transform the ideal point to the ideal point.
A transformation that transforms the ideal point to the ideal point
is called an affine transformation. The composition of two affine transformations
is an affine transformation. The inverse of an affine transformation is
an affine transformation and clearly the identity transformation is an
affine transformation, so the affine transformations are also a group under
the operation of composition (matrix multiplication).
-
Projective transformations of RP(2) with examples of transformations
previously discussed in the course.
-
Using homogeneous coordinates of points in the affine plane, we consider
these as points in RP(2). Consider the isometries of translation, rotation,
and reflection of the plane, extended to the affine plane and thus to RP(2).
Translation by <a,b>:
Rotation at (0,0) = <0,0,1>
Reflection about the X- axis:
Reflection about the line Y=X:
Central Similarities at (0,0) by a factor of M.
Reconstruction of a point on a conic from Pascal's Theorem.
The following materials are
from previous course notes and have not been incorporated into the current
course notes.
Consider lines connecting corresponding points in a pencil
of points on a line related by a projectivity (not a perspectivity) and
noticed that the envelope of these lines seemed to be a conic, a line
conic. Notice briefly the dual figure which would form a more
traditional point conic. [Also notice how line figures might be related
to solving differential equations e.g. dy/dx=2x-1 with y(0)=3 has a solution
curve determined by the tangent lines determined by the derivative: y=x^2-x+3
which is a parabola.]
In discussing the issue of whether the 5th axiom could be proven
from the other four axioms, we looked at an example of another axiom system,
with an axiom N (any pair of lines having at one point in common)
and an axiom P (given a line l and a point P not on that line there
is a line m where P is on m and m and l have no common points) which is
a version of the parallel postulate (Playfair's - not Euclid's). We gave
examples showing that the four axioms and P were possible as well as the
four axioms and N were possible. This showed that one can not prove axiom
P or N from the other four axioms since P and N are contradictory.
By a similar analysis of the axioms for the seven point geometry
we showed that it not possible prove the 5th postulate from the other four.
The analysis examines the example of the seven point geometry and notices
that by including an 8th and 9th point the resulting geometry would satisfy
the other 4 axioms.
The model we have for affine geometry still satisfies the parallel
postulate, since the ideal (infinite) points of affine geometry are not
considered as ordinary points of the geometry. However, be removing this
distinction between ordinary and ideal points and considering the geometry
that results we obtain a geometry in which there are no parallel lines.
(A projective plane.) This will be a major focus of discussion for the
remainder of the term- especially using the homogeneous coordinates to
consider points in this geometry from an analytic/algebraic approach.
-
Initial discussion considered the problems of perception and how the
position of an object in a picture can affect our judgment on its relative
size. Turning directly to the question of perspective in drawing we looked
at how an artist tries to capture the visual reality of perception by drawing
figures larger when they are closer to the eye of the beholder. We looked
at some Durer drawings showing some mechanical ways to draw accurate perspective
figures.
An examination of the problems of transferring an spatial image of
a plane to a second plane using the idea of lines of sight we arrived at
an understanding of how points in the plane would correspond to lines through
a point (the eye).
In discussing the 7 point geometry we visualized it using vertices
of a cube (besides (0,0,0)) with their ordinary coordinates in standard
3 dimensional coordinate geometry and identified the 7 points . This allowed
us to identify "lines" using the homogeneous coordinate concepts and their
relation to planes in three dimension through (0,0,0). We identified all
but one of the lines easily- the last plane has ordinary equation X + Y
+ Z = 2... but in this arithmetic for {0,1} we have 1+1=0, so 2=0 and the
vertices of that satisfy this equation in ordinary coordinates {(1,1,0),
(1,0,1),(0,1,1)} form a line as well.
We then discussed using {0,1,2} for homogeneous coordinates connected
to the arithmetic given by the tables
| + |
0 |
1 |
2 |
|
* |
0 |
1 |
2 |
| 0 |
0 |
1 |
2 |
|
0 |
0 |
0 |
0 |
| 1 |
1 |
2 |
0 |
|
1 |
0 |
1 |
2 |
| 2 |
2 |
0 |
1 |
|
2 |
0 |
2 |
1 |
The homogeneous coordinates for this set identify ordered triples, for
example: <1,0,1>=<2,0,2> and <1,0,2>=<2,0,1>. There are
27 possible ordered triples, and thus 26 when we exclude (0,0,0), and these
are each paired by the factor 2 with another triple, so there will be exactly
13 points ( and by the comparable work with lines) and 13 lines in this
geometry.
.... some details still to be reported.
-
We watched the film on central perspectivities that discussed
perspectivities and projectivities in the plane. Any perspectivity between
a pencil of points on one line and that one another line can be thought
of as a transformation. This transformation is completely determined by
the relation of two pairs of distinct points on two lines. For a projectivity
(the composition of a finite number of perspectivities) the result is that
a projectivity is completely determined by the correspondence of three
points. This result is called the fundamental theorem of projective
geometry and is taken as an axiom by M&I.
[Moved from earlier: The lab time was spent working on sketches showing
ways to understand that result of the CAROMS film about the inscribed triangles
of minimum perimeter.In Lab: Discuss some visual features such as trace
and animation and start to look at the use of coordinates. We'll do more
with coordinates, along with the use of traces and locus to see some aspects
of coordinate geometry next week.]
We also watched the film on projective generation of conics which
introduced Pascal's theorem and its converse about hexagons inscribed in
a conic and showed how to use this result to construct a conic curve passing
through any 5 points. This work was also related to projectivities between
pencils of lines. We will be considering this further in the course. After
a short break we continued using metric ideas to construct an ellipse
as a locus on sketchpad and discussed how to do a parabola as well. By
next Thursday students should construct examples of the three conics on
sketchpad using metric ideas.
-
Okay... just a quick recall of some of what we covered:
-
Now that we've established how to connect homogeneous coordinates to
a projective line using harmonics we continued by looking at some more
algebraic projective lines- with coefficients of Z2,
Z3,
and
Z5, we saw that these projective lines would have 3,4, and
6 points respectively, corresponding to the 2,3,and 5 ordinary points and
one ideal point. This lead to
-
A discussion of using the complex numbers, C, and the geometry
of CP(1). The ordinary points on this "line" can be visualized as
a plane or all the points of a sphere except one- which corresponds to
the north pole or the point at infinity. [This was compared to the visualization
of RP(1) as a circle.]
-
The discussion turned to algebraic projective transformations of RP(1).
When these leave the ideal point fixed they are called affine transformations
and form a group under composition. We showed that an affine transformation
has a matrix of the form
and therefore T(Px)=Pax+b. Thus an affine transformation of the projective
line is a dilation/reflection followed by a translation.
-
We looked at the idea of establishing the idea of a distance between
ordinary points using absolute value-
d( <a,b>,<c,d>) = |a/b - c/d|. We showed
this is well defined and then discussed what the isometries for this distance
would be. After some analysis we saw that this would mean that in the matrix
of an isometry |a|=1, so a=1 or a=-1. I.e, the matrix is
or that T(Px)=P+/-x+b. Thus an isometry of RP(1) is a reflection followed
by a translation.
-
We also looked further at Pascal's theorem and its planar dual Brianchon's
theorem. Next class we'll prove Brianchon's theorem using an elliptic hyperbaloid.
-
Today we did more on algebraic projective transformations of CP(1).
This meant first examining what it meant for these to be affine, and then
the geometry of addition and multiplication of complex numbers. Addition
of a constant complex number corresponded to a translation. Multiplication
by a constant corresponded to a dilation using the absolute value of the
complex number for the factor and a rotation by the angle the corresponding
vector mad with the positive real axis. We also saw how affine transformations
could preserve the distance between complex numbers if |a|=1 where
a is a complex number, so a can be thought of a point on the unit circle
and multiplication by a corresponds to a rotation. So affine transformations
of CP(1) are rotations followed by translations of the plane of
complex numbers. Notice these are all orientation preserving transformations.
so any reflection of the plane is not an isometry in this geometry. We
looked briefly at another transformation that was a reflection, namely
complex conjugacy, T(a+bi)=a-bi. This is not an isometry in this geometry
because it is orientation reversing.
We spent the remainder of the lecture time going over the proof of
Brianchon's Theorem using the proof of Hilbert and Cohn-Vossen based on
hexagons lying on the surface of an elliptic hyperbaloid (which is a ruled
surface).