Desargues' Theorem
Notes by M. Flashman
Based on proof found in Hilbert&Cohn-Vossen's
Geometry
and The Imagination.
Desargues' Theorem: (in projective 3 space). If two non co-planar
triangles ABC and A'B'C' are perspectively related by the center O, then
the points of intersection X=AB#A'B'; Y=AC#A'C' ; and Z=BC#B'C' all lie
on the same line.[# is used here to indicate the intersection of the lines.]
Proof: The intersection of the planes determined by the two triangles is
a line. This line has the points X, Y, and Z on it, because these points
must lie on the intersection of the two planes.
In a "projective spatial geometry" any pair of distinct planes would
intersect in a line. EOP.
Notice that if we use a central projection of the spatial configuration
of lines and vertices from the spatial Desargues' Theorem we have a planar
configuration of lines and vertices, satisfying the same hypotheses and
illustrating the same conclusion. This basic idea of projecting a result
from 3-space onto the plane is used in the proof of the planar version
of Desargues' Theorem as provided in the book Geometry and The Imagination
by Hilbert and Cohn-Vossen.
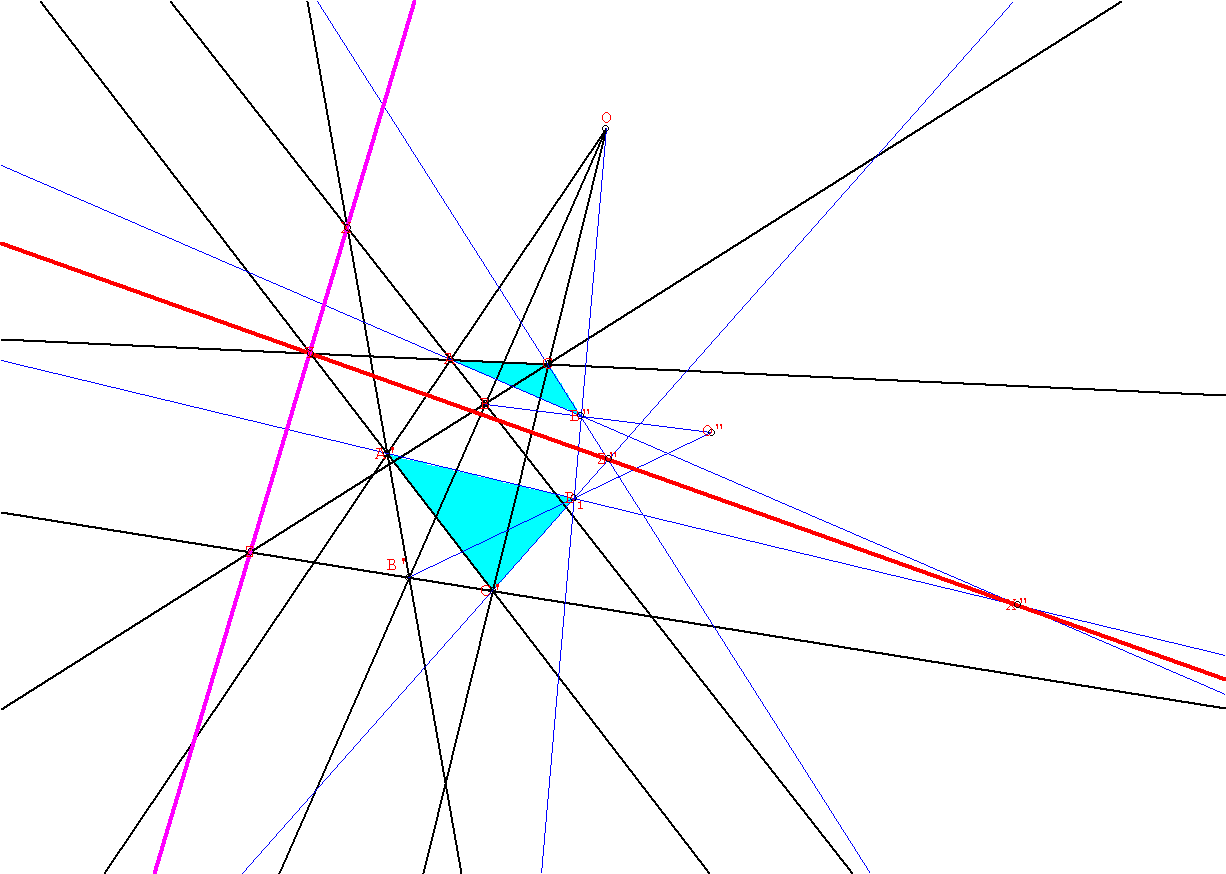
Desargues' Theorem: (in the (projective) plane). If two coplanar
triangles ABC and A'B'C' are perspectively related by the center O, then
the points of intersection X=AB*A'B'; Y=AC*A'C' ; and Z=BC*B'C' all lie
on the same line.
Proof: Choose O* not in the plane ABC. Choose B* a point on BO*.
Let B'* denote the intersection of O*B' with OB* in the plane OBO*.
Now triangles AB*C and A'B'* C' are perspectively related
in space by the center O.
So by Desargues' Theorem in space, the points X*=AB*#A'B'*;
Y*=AC#A'C' ; and Z*=B*C#B'*C' all lie on the same line l* in
space which does not pass through the point O*.
Now the plane determined by l* and O* meets the plane ABC
on a line l.
Consider that O*X* #ABC = {X}, Y* = Y and O*Z* #ABC = {Z}.
Thus the three points X, Y and Z all lie on the line l.
EOP.