I. What do we understand by the number 7?

1. Distinguish the number from the numeral:
1. Symbols , words, and other designations.

Examples of numerals for the number seven.
• Various Arabic numerals


 
• Roman Numerals I, II, III, IV, V, VI, VII
• Egyptian numerals


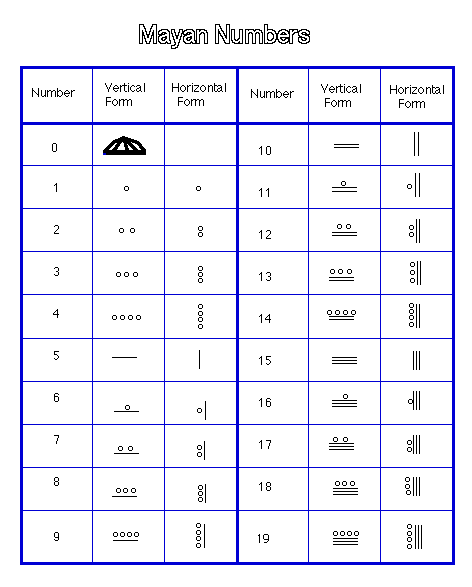
• Mayan Numerals


• Binary Notation: 1,10,11,100,101,110,111
• Different Languages: ....
 
 
2. What defines the number 7? Webster's definitions.

Encyclopedia Britannica .
 
2. Meeting the number 7 in some common places.
1. On a calendar.
2. On a color wheel.
3. In games and sports.
• 7 fielders in baseball (not part of the "battery").
• 7 players in indoor soccer
• 7 line players in football
• 7 card poker games (7 card stud and Texas Hold'em)
4. In mystical/religious writings
• lucky 7
• 7 deadly sins.
5. In music and dance:
• The seven notes of a diatonic scale.
• The dance of the seven veils.
6. In literature:
• The House of Seven Gables
• Seven Samurai
• Seven Brides for Seven Brothers
• Snow White and the Seven Dwarfs
7. In geography:
• The seven seas.
• The seven (?) continents.
• The seven wonders of the world.
8. In committee  organization:

The HSU Math department has 7 standing committees.
                                          Personnel

             Kieval Lecture                                Scholarship/prizes

                                          Curriculum
 

Technology                          Statistics                Teacher Preparation
 

 

3. Definitions of the number 7.
1. By itself.
• A cardinal number of a set.
• An ordinal number from an ordered set.
2. By its  unique properties:

The unique number such that .....
3. By recognizing its qualities in a variety of contexts.
• Common
• Mathematical

1. Musical structures.

An atonal chord structure.
2. Committee structures.

A committee structure.
3. Scheduling structures.

A design for scheduling classes?
4. Color Wheel/Triangle. (show with GSP)

 

1. A Geometric structure
• A graphical geometry.
• Visualizing this geometry. (GSP)
2. A Geometric structure
• A triangular geometry.
• Visualizing this geometry. (GSP)
3. Some projective geometric ideas based on a focus.
• A line through the focus appears as a point.
• A plane through the focus appears as a line.
• Two points determine a plane including the focus.
• Any two planes through the focus determine a line through the focus.
• Definitions:

A line through the focus is called a projective point (a P-point) and
A plane through the focus is called a projective line (a P-line).

A projective plane is the geometric object made up of the collection of P-points and P-lines.

• In a projective plane,

any two P-points determine a unique P-line and any two P-lines determine a unique P-point.
4. Some algebraic descriptions of lines and planes in 3 dimensions.
• A plane through the origin has an equation of the form Ax + By + Cz  = 0, where [A,B,C] is not [0,0,0]. The triple [uA,uB,uC] will determine the same plane as long as u is not 0.

For example, [1,0,1] determines the plane with equation X + Z = 0.
• A line through the origin has the equation of the form (X,Y,Z) = (a,b,c) t where (a,b,c) is not (0,0,0).The triple (ua,ub,uc)will determine the same line as long as u is not 0.

For example, (1,0,-1) deteremines the line with equation (X,Y,Z)=(1,0,-1)t.
• A P-line has an equation of the form Ax + By + Cz  = 0, where [A,B,C] is not [0,0,0].

The triple [uA,uB,uC] will determine the same plane as long as u is not 0.

We'll call [A,B,C] homogeneous coordinates of the P-line.

For example, [1,0,1] are homogeneous coordinates for the P-line determined by the plane with equation X + Z = 0.

• A P-point has the equation (X,Y,Z) = (a,b,c) t where (a,b,c) is not (0,0,0).The triple (ua,ub,uc) will determine the same line as long as u is not 0.

We'll call <a,b,c> homogeneous coordinates of the P- point. 

For example, <1,0,-1> are homogeneous coordinates for the P-point determined by the line with equation (X,Y,Z) = (1,0,-1) t.

• A P-point lies on a P line or a P -line passes through the the P-point if and only if  Aa+Bb+Cc= 0 where [A,B,C] are homogeneous coordinates for the P-line and <a,b,c> are homogeneous coordinates for the P-point.

For example, the P-point <1,0,-1> lies on the P-line [1,0,1].
• NOTE: All of the discussion works as long as the symbols A,B,C, a,b, and c represent elements of a field, that is, a set with two operations that work like the real or rational numbers in terms of addition and multiplication.
5. A field with two elements:   F₂ = {0,1}.

 

+	0	1			x	0	1
0	0	1			0	0	0
1	1	0			1	0	1

 

A projective plane using F₂ has  exactly 7 points:

<0,0,1>, <0,1,0>, <0,1,1>,<1,0,0>,<1,0,1>,<1,1,0>,<1,1,1>.  

A projective plane using F₂ has exactly 7 lines:

[0,0,1], [0,1,0], [0,1,1], [1,0,0], [1,0,1], [1,1,0], [1,1,1].  
 

This projective plane satisfies the geometric structure properties.

Lines\Points	<0,0,1>	<0,1,0>	<0,1,1>	<1,0,0>	<1,0,1>	<1,1,0>	<1,1,1>
[0,0,1]		X		X		X
[0,1,0]	X			X	X
[0,1,1]			X	X			X
[1,0,0]	X	X	X
[1,0,1]		X			X		X
[1,1,0]	X					X	X
[1,1,1]			X		X	X

 

We can visualize the projective plane  using F₂ using 7 points of the unit cube in ordinary 3 dimensional coordinate geometry. (GSP)

    THE END.