Math 105 Fall, 2005

Assignments-SUBJECT TO REVISION!
#9: Submit by 12-15 :#8: 11-17  to 12-1 #7: 11-3  to 11-15
 #6: 10-25  to 11-3
 #5: 10-11  to 10-25 #4: 9-27  to  10-11
 #3: 9-20  to  9-29
 #2 : 9-8 to 9-20
 #1:9-1 to 9-8
Resource List
Final Assignment #9.
[May be submitted until 12-15]
Over the term we have covered many topics in class and through the readings. Choose two topics we have studied for examples in writing a paper (1-3 pages) discussing one of the following statements:
     A. The study of visual mathematics in two dimensions has much in common but also some noticable differences with its study in three dimensions.
     B. The amazing thing about mathematics is how it is able to turn even the simplest things into abstractions and can make the subtlest of concepts clear through a figure.
Assignment #8: November 17 - November 29 .

Read Devlin:   Pp. 74-79 (Zeno's Paradoxes and the infinite.)
                         Pp. 115-119 (Conics and cartesian geometry)
                         Pp. 129 - 133;134-138 (Projective Geometry)
                         Pp. 106-109 (Euclid's Axioms)
                         Pp. 122-129 (Non-Euclidean Geometries)

   Exercises/Activities: To be collected on Tuesday, November 29  

I.  Look up "Zeno's paradoxes" in the Encyclopedia (Britannica). Draw a figure that illustrates the paradox of Achilles and the Tortoise. Describe an common situation today to which Zeno's argument about Achilles and the Tortoise could be applied. Using your situation, discuss where the accumulation of small and infinitely divisible intervals is incorrectly compared with the accumulation of equally sized intervals.

Coordinate geometry is a tool used in intermediate algebra courses to investigate the conic curves. Recall the basic idea is that a point with coordinates (x,y) will lie on a curve in the coordinate plane if and only if the numbers x and y make an equation determining the curve true.
   For example, a circle with center (0,0) and radius 5 is determined by the equation X2 + Y 2 = 25. We can check that the point with coordinates (3,4) is on the circle by verifying that 3 2 + 4 2 = 25.

II. Each of the following equations determines a conic curve.
Plot 8 points for each equation on a standard rectangular coordinate graph. Connect these points with straight line segments to give a polygon that will approximate the curve.
                      
    a. 4X 2 + Y 2 = 25     [an ellipse]
    b.   X  2 - Y  2 =  9  [an hyperbola]
    c.  X 2 - Y   =  4     [a parabola]

III. Draw a system of coordinates on the projective plane with the horizon line and lines for X=1,2,3,4,and,5 and Y=1,2,3,4,and 5 as well as the X and Y axes.
For each of the previous equations, plot 6 points on a projective coordinate plane that correspond to 6 of the 8 points plotted previously on the standard plane. Connect these points with straight line segments in the projective plane.

IV. Discuss the following statements, drawing figures to illustrate your remarks:
In the projective plane, any conic curve will look like an ellipse. The main distinction between the curves is the number of ideal points that lie on the conic.
An ellipse will have no ideal points, a parabola will have one ideal point, and an hyperbola will have exactly two ideal points.
Assignment #7: November 3 -15 .

Read Devlin:    pp. 138-141 (Dimension)
                          pp. 179-182.(The Moebius strip, orientability)
                          pp. 182-186, 187(1st paragraph.) (Surfaces)

Exercises/Activities: To be collected on Tuesday, November 15

I.Cubes.
See page 140 in Devlin.
Sketch the face-on view of the cube and the hypercube.
Label the coordinates of your sketches.
Determine the number of vertices, edges, faces, and cubes in the hypercube .

II. Maps.
Find three different types of world maps. Copy each by tracing or photo or by “cut and paste”. Write a short report (about one page) describing how both the poles and the global coordinates determined by longitude and latitude are visualized on each planar map.

III.Surfaces.
A. Describe 5 physical objects that have surfaces that are topologically equivalent to a (one hole) torus. Bring one example to class on Thursday.
B. Describe 2 physical objects that have surfaces that are topologically equivalent to a torus with two or more holes. Bring one example to class on Thursday.

IV. Coloring the Torus and the Moebius Strip.
A. Draw a map on the Torus with 5 regions, each having a border with the other 4.
B. Draw a map on the Moebius Strip with 5 regions, each having a border with the other 4.

V. The Fourth Dimension.
The fourth dimension can be used to visualize and keep track of many things that involve four distinct qualities that can be measured in some fashion.

A. For example, a 13 card bridge hand can be thought of as a point in four dimensions where the coordinates represent the number of cards of each suit present in the hand. In this situation the point with coordinates ( 2,4,6,1) might represent a hand with 2 clubs, 4 diamonds, 6 hearts and 1 spade.
Suppose a bridge hand is represented by the point with coordinates (x,y,z,w).
Explain why x+y+z+w=13.

B. Describe another context where four dimensions can be used in representing the context.
Assignment #6: October 25-November 3

I.Group the following letters, as printed on this page, together in different classes determined by whether they are topologically equivalent. [It is up to you to determine the appropriate classes.]
A B C D E F G H I J K L M N O P Q R S T U V W X Y Z

II (A recycled problem) We are still trying to describe the cube to a Flatlander, this time using the transformation of the framework of the cube onto the plane by central projections.
Show the image of the cube on the plane under the following projection situations:
A.  One square of the cube is in Flatland and the center of projection is above the cube directly over the center of the cube.
B.  Only one edge of the cube is in Flatland and the center of projection is above the cube directly over the center of the edge in Flatland.
C.  One square of the cube is in Flatland and the center of projection is higher than the cube and not directly over any part of the cube.

Assignment #5: October 11-October 25
Read Devlin:    pp. 174 - 175; 176 - 178 (Topology, Networks, and Euler's formula)
                        pp. 188-189 (The Four Color Problem)

Exercises/Activities: To be collected on Tuesday, October 25

  
I. Networks and Euler's Formula.
A. A message has arrived from the planet Yxo describing a road network connecting 8 major cities on the planet. The message claims that the network has exactly 15 edges and divides the planet into 6 separate regions. Based on Euler's formula discuss the following statement:
Either the planet is not spherical in general shape or the information is incomplete.
B. A planar graph has been drawn by a designer using exactly 12 edges and 8 vertices. How many regions are created by this graph?   Explain how you arrived at your count.
Draw a graph that is evidence that such a graph is possible.

II. Networks As Models.
Choose a floor in a building on the Occidental campus.
A. Describe the layout of this floor as a network. Include a sketch of the network together with a legend explaining the correspondence of vertices and edges to the building's features.
B. Suppose there is an electrical blackout at night. Discuss how you could use your network description to help find your way through the floor to leave the building.
C. Compare the situation of the floor layout network to Devlin's London Underground Network. How are their uses alike? How do they differ?

III. Projections and  Cut-up Flattened Semi-Regular Polyhedra Frameworks.
Draw  cut-up flattened frameworks or networks for the following semi-regular polyhedra. Iidentify the edges that would be pasted together to reassemble the framework in space.
A. The truncated cube.         B. The truncated octahedron.

Assignment #4: September 27 - October 11
Tessellation Thursday, October 6th.
On Thursday, October 6th,  wear to class clothing that has a tiling (wallpaper) pattern on it.

Read Devlin:  pp. 112-115 (Euclid- inscribed angles in semicircles,Platonic solids, Plato, and Kepler)
pp 129-132 (Introduction to the geometry of projection)
Plato's Cave Metaphor- online.

Exercises/Activities: To be collected on Tuesday October 11.
I.Creating new tessellations by modifications.
Create a tessellation of curved figures following the ideas from the activity of September 29th applied to the regular tiling of the plane by (1) hexagons and (2) equilateral triangles.

II. The Sphere has brought the Cube to visit his new friend in Flatland. Describe two possible successions of different planar shapes the Cube might  appear as while passing through Flatland.
Draw the sequences as they would be seen in Flatland.        

III. Projections: Casting Shadows on Flatland.
The sphere is still trying to explain some of the features of the torus to a Flatlander. This time the sphere has decided to show the Flatlander different shadows that are cast by the projection of the torus onto Flatland.
A. Draw three different shadows that the torus could cast.
B. Do you think it is possible to make a torus that would cast a shadow on Flatland that completely covers a circle and its interior? If so, describe some of the features of such a torus. If not, give some reasons for your belief. In other words, is it possible that a Flatlander might mistake a torus for a sphere based on the shadow it casts?

IV. We are still trying to describe the cube to a Flatlander, this time using the transformation of the framework of the cube onto the plane by central projections.
Show the image of the cube on the plane under the following projection situations:
A.  One square of the cube is in Flatland and the center of projection is above the cube directly over the center of the cube.
B.  Only one edge of the cube is in Flatland and the center of projection is above the cube directly over the center of the edge in Flatland.
C.  One square of the cube is in Flatland and the center of projection is higher than the cube and not directly over any part of the cube.

V.Plato and Shadows: The Greek philosopher Plato describes a situation where a person lives in a cave and can only perceive what happens outside the cave by observing the shadows that are cast on the walls of the cave from the outside.

Write a brief essay discussing a situation in the contemporary world where indirect experiences are used to make observations. How are the observations made? How are they connected to the actual situation? Do you think the inferences made from the observations are always accurate? [3 or 4 paragraphs should be adequate.]

 

 Assignment #3: September 20 - September 29

Read Devlin:   pp. 153-157 (lattices and sphere packing);
pp. 163-164 (wallpaper patterns);  pp. 144-150 (Symmetry Groups again!); pp.165-170 (tiling again!).
Exercises/Activities: To be collected on Thursday September 29.

I. Lineland Paper:
    Imagine you are a Flatlander talking to a Linelander. Write an explanation of symmetry to a Linelander from the point of view of a Flatlander. Discuss and illustrate the kinds of symmetry that are possible in Lineland. Which Flatland symmetries (if any) would you associate with Lineland symmetries? Explain the association briefly.
Here are some terms you might use in your discussion: Reflection  Rotation  Translation  Orientation

II. Dual tessellations and symmetry.
    A. Draw the tessellation of the plane with equilateral triangles. Place a point in the center of each triangle and connect these points with a line segment when they are in adjacent triangles. Describe the symmetries of the resulting tiling of the plane.
    B. Draw the semiregular tessellation of the plane that uses squares and octagons.[See  Devlin p.166.]  Place a point in the center of square and octagon and connect these points with a line segment when they are in adjacent figures. Describe the symmetries of the resulting tiling of the plane.

III. [This problem may be done with a partner]. Symmetry group of a square.
    There are 8 symmetries for any square.
    A. Provide a sketch of the square and illustrate the 8 symmetries as in the triangle example. [See the class notes.]
    B. Determine the "multiplication table" for the group of symmetries of the square. [See Devlin page 150] 

Assignment #2: September 8 - September 20.

Read Flatland:    Part II.
Read Devlin:    pp. 144-150 (Symmetry Groups); pp. 165-169 (Tiling).
Exercises/Activities: To be collected on Tuesday September 20.

I. Using the seven pieces of the tangram puzzle create
A. A rectangle   and B. A right triangle.

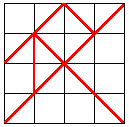
II. Suppose that the square made using the seven tangram pieces has a side of length 4.

A. Find the length of the sides of each of the seven pieces.
B. Find the area of each of the seven pieces.

III. Classifications by reflection symmetries:
   It is often useful to classify visual objects by their symmetries. For example, the letter "T" as it appears on this page has only a reflection symmetry determined by a vertical line, whereas the letter "I" has two reflection symmetries and "J" has no reflection symmetry.

Group the following letters, as printed on this page, together in different classes determined by the number of reflection symmetries they have. [It is up to you to determine the appropriate classes.]
A B C D E F G H I J K L M N O P Q R S T U V W X Y Z

IV. Assume the following pattern is extended indefinitely on the plane. This pattern has many lines of reflectional symmetry.  Draw in all the lines of symmetry using colored pencils so that any two lines with the same color are parallel and any two lines that are parallel are colored with the same color.


V. Find (or create) a graphic design (in advertisements, logos, or icons) that
(i) has exactly one reflection symmetry, (ii) has exactly two reflection symmetries, and (iii) has exactly three reflection symmetries.
 Indicate and discuss the symmetry line(s) in each separate design.
Assignment #1: September 1 - September 8.

Read Flatland. Introduction, Preface, and Part I.
(Activity and assignments on Flatland will follow next week.)

Read Devlin:    Prologue, pp. 1-7. 
            Chapter 1, Greek Mathematics, pp. 14 - 18, 21, and 31.

Exercises/Activities: To be collected on Thursday, September 8.

I. Ask your adviser or an instructor  you know in your major the following two questions:
    A. How do you define mathematics?  (or What is mathematics?)
    B. Can you give one example of how mathematics is used in your discipline? Ask for an explanation of the response.

Report the responses to these two questions and relate them to Devlin's treatment of question A.
[Two paragraphs are sufficient.]
   

II. [See Devlin, p.21]
Draw a rectangular figure arranging 30 discs into 5 rows.
    A. Draw a straight line through the rectangle that divides the discs into two groups each with 15 discs.
    B. According to the formula on Devlin page 21, using n = 5, 
    1 + 2 + 3 + 4 + 5 = 5 (5+1) / 2.
Explain the relation of this equation to the arrangement of the 30 balls in part A.
    C. Describe an analogous figure to explain why
    1 + 2 + 3 + ⋯ + 98 + 99 + 100 = 10,100/2 = 5,050

Other resources: Flatland is available on the web.
Over 30 proofs of the Pythagorean theorem!
Many Java Applets that visualize proofs of the Pythagorean Theorem
Tangram Introduction
Japanese Site with Tangram Puzzles on-line
Web references related to scissors congruence- dissections.
 

Resource List for Portfolio Entries

The following list contains suggestions  for finding resources as well as the names of resources that may be used for one or more portfolio entries. Before reading an article in one of these resources thoroughly it is advisable to scan it quickly to see that it contains something of interest to yourself. Your portfolio entry can report on the content of your reading, illustrate it by examples, and/or follow up on it with your own response and creativity.

The content of the portfolio entry should relate specifically and directly to some visual mathematics. Personal observations , philosophical musings, and aesthetical judgments are not adequate connections to something visual by themselves to qualify as mathematical content.

These articles may also be useful in developing a deper level of understanding on a topic which will suppport your term project. I will add to this list as the term progresses.