Suppose P is on l and PN intersect C = {Q}; Q' is on C so that Q'Q is parallel to ON; and {P'} = NQ' intersect l.
TEXTS: Fundamentals of Geometry by B. Meserve and J. Izzo,
A.W. (1969) -
ON LINE with HSU ONCORE
through Blackboard.
The
Elements by Euclid, 3 volumes, edited by T.L. Heath, Dover
(1926)
Proof in Geometry by A.I Fetisov, Mir (1978)
Here's Looking at Euclid..., by J.Petit, Kaufmann (1985).
Flatland By E. Abbott, Dover.
| Week | Monday | Wednesday | Friday | Reading/Videos for the week. | Problems
Due on Wednesday of the next week | Math 480 Lab Assignments |
|---|---|---|---|---|---|---|
| 1 | 1/19 No Class | 1/21 1.1 Beginnings
Lab: Intro to Geometer's Sketchpad/ Wingeom |
1/23 |
M&I:1.1,
1.2
E:I Def'ns, etc. p153-5; Prop. 1-12,22,23,47 A:.Complete in three weeks |
Due: 1/28 M&I p5:1-8,11 | |
| 2 |
1/26 The Pythagorean Theorem |
1/28
1.2 Equidecomposable Polygons |
1/30Finish proof of EP. More on Constructions Isometries 1.1 Def'ns- Objects 1.2 Constructions |
M&I
1.2, 1.3
E: I Prop. 16, 27-32, 35-45. |
Due: 2/4
M&I:
p10:1,2,5,10,11-13 Prove:The line segment connecting the midpoints of two sides of a triangle is parallel to the third side and is congruent to one half of the third side. |
Lab Exercises 1: Due: 2/3 Construct a sketch with technology of 1. Euclid's Proposition 1 in Book I. 2. Euclid's Proposition 2 in Book I. 3. One "proof" of the Pythagorean Theorem. |
| 3 | 2/2 1.3 Geometry: Constructions and numbers | 2/41.4 Continuity | 2/6 Beather: Start: Transformations - Isometries |
M&I
1.3,1.4
E: III Prop. 1-3, 14-18, 20, 21, 10 F. Sect. 11, 25, 31 Watch Equidecomposable Polygons on -line Notes from Prof. Biles on trig. |
Due: 2/11
Changed M&I: p17:5, 8-11 p11: 16-19, 24, *27 Due:2/11changed Problem Set 1 REVISED: Now Due 2/16 No reading report for 2/16! | Lab Exercises 2: Due by 2/10.
Do Construction 3, 4, 6, 7, and 8 from Meserve and Izzo Section 1.2. BONUS:Show how to "add" two arbitrary triangles to create a single square. |
| 4 | 2/9 Inversion, Orthogonal Circles | 2/11 Odds and ends. | 2/13 Transformations - Isometries. Coordinates. |
M&I:1.5,
1.6,
2.1
E: V def'ns 1-7;VI: prop 1&2 F. Sect. 32 |
Due: 2/11
Changed M&I: p17:5, 8-11 p11: 16-19, 24, *27 Due:2/11changed Problem Set 1 REVISED: Now Due 2/16 No reading report for 2/16! |
Lab Exercises 3: Due 2/17.
1. Construct a scalene triangle using Wingeometry. Illustrate how to do i) a translation by a given "vector", ii) a rotation by a given angle measure, and iii) reflections across a given line.. 2. Create a sketch that shows that the product of two reflections is either a translation or a rotation |
| 5 |
2/16 More Isometries: Coordinates and Transformations
|
2/18 Isometries / coordinates/ classification |
2/20 Finish Classification of Planar Isometries. |
M&I:
2.1, 2.2,
E:IV Prop. 3-5 |
Due 2/25: M&I: p23: 9,10 (analytic proofs) M&I:1.6:1-12,17,18 Problem Set 2 |
Lab Exercises 4: Due 2/24.
1. Draw a figure showing the product of three planar reflections as a glide reflection. 2. Draw a figure illustrating the effects of a central similarity on a triangle using magnification or dilation that is a) positive number >1, b) a positive number <1, and c) a negative number. |
| 6 | 2/23 Isometries and symmetries .... Begin Affine Geometry | 2/25 Inversion and Affine Geometry (planar coordinates) | 2/27 Seeing the infinite |
M&I: 2.1,2.2 | Due
3/5 Problem Set 3 (Isos Tri) [4 Points for every distinct correct proof of any of these problems.] |
Lab Exercises 5: Due 3/2 .
Construct the inverse of a point with respect to a circle a) when the point is inside the circle; b) when the point is outside the circle. |
| 7 | 3/1 More on the Affine Line. | 3/3 More on Affine geometry- Homogeneous coordinates and visualizing the affine plane. | 3/5Visualizing the affine plane. | M&I: 3.1,3.2, 3.5 | Due 3/10 M&I: 3.5: 1,3,4,5,10,11 |
Lab Exercise 6:
Due 3/9 1.Draw sketches for each of the following triangle coincidences: 1. Medians. 2. Angle Bisectors. 3. Altitudes. 4. Perpendicular Bisectors. 2. Given a circle O and two interior points A and B, construct an orthogonal circle O' through A and B. 3. Draw two intersecting circles O and O' and measure the angle between them. |
| 8 |
3/8 More on Homogeneous coordinates for the plane. | 3/10 Begin Synthetic Geometry [Finite] | 3/12 Homogeneous Coordinates with Z2 and Z3 More on Finite Synthetic Geometry and models. |
M&I:3.6, 3.4,3.7 | Due: 3/24 Problem Set 4 3.6: 3,7-15 3.7: 1,4,7,10,13 | Lab Exercise 7: Due 3/23
.
1. Inversion: Investigate and sketch the result of inversion on lines and circles in the plane with a given circle for inversion. When does a line invert to a line? When does a line invert to a circle? When does a circle invert to a line? when does a circle invert to a circle? Show sketches where each case occurs. [ Remember the inverse of the inverse is the original figure.] 2. Use inversion with respect to the circle OP to invert <BAC to <B'A'C'. Discuss briefly the effects of inversion on angles. 3. Draw a sketch of the affine plane showing the horizon line and label the lines X=1,2,-1, Y= 1,2,-1 and points (1,2) and (2,-1). |
| 9 Spring break | 3/15 No Class | 3/17 No Class | 3/19 No Class |
|||
| 10 | 3/22 Axioms, consistency, completeness and models. A non-euclidean universe. |
3/24 Algebraic-projective geometry: Points and lines. Spatial and Planar Desargues' Theorem Proof of Desargues' Theorem Begin Synthetic Projective Geometry |
3/26 -Projective Geometry -Visual/algebraic and Synthetic..Axioms 1-6 Projective Planes. . | M&I:4.1, 4.2, 4.3, 2.4 | Due 4/7. M&I:4.1:7,15,16; Prove P6 for RP(2); 4.2: 2,3, Supp:1 4.3: 1-6, Supp:1,5,6 | Lab Exercise 8: Due 4/6 Draw a sketch for Desargue's theorem in the plane. |
| 11 | 3/29 More on the axioms of Projective Geometry.RP(2) as a model for synthetic geometry.
|
3/31 No Class.
CC Day. |
4/2
Proofs of some basic projective geometric facts. Triangle Coincidences (Perpendicular Bisectors- the circumcenter) |
M&I:4.1, 4.2, 4.3, 2.4 | No Lab this week. |
|
| 12 | 4/5 Duality Theorem and Desargues. | 4/7Conic Sections.
Pascal and More Duality |
4/9 Complete quadrangles Postulate 9.
Projective transformations. Perspectivities and Projectivities. |
M&I:
4.5,4.6(p94-97).4.7, p105-108 (Desargues' Thrm) |
Due : 4/21 M&I:4.5:2; 4.6:7,8,9; 4.7:4,7 4.10: 4,5,9,10 [Prove P9 for RP(2),optional] | Lab Exercise 9: Due 4/13
Pascal's configuration: Hexagons inscribed in conics. Points of intersections of opposite sides lie on a single line. Construct a figure for Pascal's configuration with a) an ellipse , b)a parabola, and c) an hyperbola. |
| 13 | 4/12 Conics
Pascal's Theorem ? More on coordinates and transformations. |
4/14 Projectivities. Perspective | 4/16Transformations of lines with homogeneous coordinates. | 4.10, 5.4, 2.4
4.11, |
Lab Exercise 10: Due 4/20 A.1. Construct a sketch showing ABC on a line perspectively related to A'B'C' on a second line with center O. 2. Construct a sketch of ABC on a line projectively (but not perspectively) related to A'B'C' on a second line. Show two centers and an intermediate line that gives the projectivity. B.1'. Draw a dual sketch for the figure in problem 1. 2'. Draw a dual sketch for the figure in problem 2. C.3.Construct a sketch of ABC on a line projectively (but not perspectively) related to A'B'C' on the same line. Show two centers and an intermediate line that gives the projectivity. D 3'. Draw a dual sketch for the figure in problem 3 |
|
| 14 | 4/19 Projectivities in 3 space: More on Projective Line Transformations
with Coordinates. |
4/21 Harmonic sets: uniqueness and construction of coordinates for a Projective Line, Plane, Space. | 4/23 More on Transformations, Coordinates and Harmonic sets. . |
5.1,5.4 | Due: 4/28 M&I: 4.10:1,3,6,7; 5.1:5; 5.4:1-8,10; 5.5: 2,3,7 | Lab Exercise 11: Due 4/27 1.Draw a sketch showing H(AB,CD) and H(CD, AB). 2. Draw a sketch that shows that if H(AB,CD) and H(AB,CD*) then D= D*. |
| 15 | 4/26 Matrices for familiar Planar Projective Transformations. |
4/28 Conics revisited. Inversion and the final exam. |
4/30 Quiz #3 Inversion. |
5.1,5.2, 5.3,5.5, 5.7, 6.1, 6.2 | Lab Exercise 12: Due 5/4 Use five points and Pascal's Theorem to construct a conic. | |
| 16 | 5/3
Projective generation of conics Pascal's and Brianchon's Theorems. |
5/5
Inversion angles, circles and lines. |
5/7 The Big picture in Summary. Student Presentations |
6.4, 6.6, 6.7 |
DEFINITIONS: A figure C is called convex if for any two
points in the figure, the line segment determined by those two points is
also contained in the figure.
That is, if A is a point of C and B is a point of C then the line segment
AB is a subset of C.
If F and G are figures then F int G is { X : X in F and
X in G }.
F int G is called the intersection of F and G.
If A is a family of figures (possibly infinite), then int
A
= { X : for every figure F in the family A, X is in F }.
int A is called the intersection of the family A.
-----------------------------------------------------------------
1. Prove: If F and G are convex figures , then F int G is a convex figure.
2. Give a counterexample for the converse of problem 1.
3. Prove: If A is a family of convex figures, then int A is a convex figure.
4. Prove: The line segment RS is convex. [ Refer to M & I pg.2.]
1. Suppose n is a natural number. Given P0 and P1 , prove by induction that you can construct with straight edge and compass (SEC) a point P sqrt(n) which will correspond to the number sqrt(n) on a Euclidean line.
2. Suppose we are given P0, P1, and P a where P a corresponds to the real number a>0. Give a construction with SEC of a point Psqrt(a) which will correspond to the number sqrt(a) on a Euclidean line.
3. Given points P0, P1, Px, and Py on a Euclidean line corresponding to the real numbers x>0 and y>0, give constructions with SEC for the following points.
| a) P x + y | b) P x - y | c) P x *y | d) P 1/x |
5. Suppose that d(A,B) = d(A',B') and that l is the perpendicular bisector of the line segment AA'. Let B'' be the reflection of B across l, i.e., B''= Tl(B). Prove that if B' is not equal to B'' then A' lies on the perpendicular bisector of the line segment.
1. Prove: Two of the medians of an isosceles triangle are congruent.
2. Prove: If two of the medians of a triangle are congruent then the triangle is isosceles.
3. Prove: The angle bisectors of congruent angles of an isosceles triangle are congruent.
4. Prove: If two of the angle bisectors of a triangle are congruent then the triangle is isosceles.
1. Use an affine line with P0 , P1 , and Pinf given. Show a construction for P1/2 and P2/3.
2. Use an affine line with P0 , P1 , and Pinf
given. Suppose x > 1.
Show a construction for Px2 and Px3 when
Px is known.
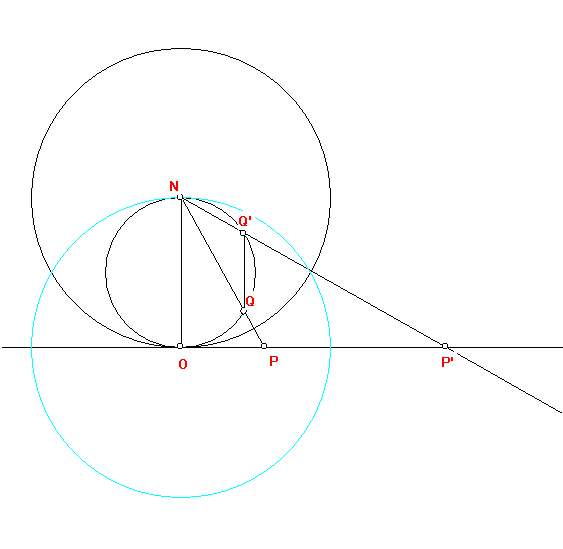
3. D is a circle with center N tangent to a line l at the point
O and C is a circle that passes through the N and is tangent to l at
O as well.
Suppose P is on l and PN intersect C = {Q}; Q' is on C so that
Q'Q is parallel to ON; and {P'} = NQ' intersect l.
Prove: a) P and Q are inverses with respect to the circle D.
b) P' and Q' are inverses with respect to the circle D.
c) P and P' are inverses with respect to the circle with center at
O and radius ON.
4. Suppose C is a circle with center O and D is a circle with O
an element of D.
Let I be the inversion transformation with respect to C.
Prove: There is a line l, where I(P) is an element of l for all P that are elements of D -{O}.