MATH 240 Introduction to Mathematical Thought
Fall, 2006 Class Summaries
-
Monday, Aug. 21. First class: Review of the structure
of the course.
- Keys to success in this course:
- Active and regular participation.
- Open to new ways of organizing your thinking.
- Communicating often and regularly with others both in oral and written forms.
- Aim for EXCELLENCE!
- Keep a sense of humor .
- Wednesday Aug. 23:
Activity Discussion (5-10 minutes):
- With a Partner discuss how Figure 1 can be used to "prove" : x + 1/x >= 2.
One partner(A) should write the "proof" while the other partner(B) checks to see the writing fully reports their understanding.
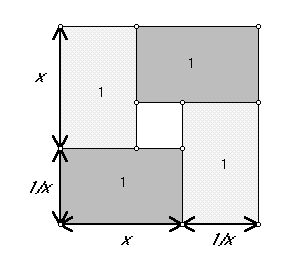 Figure 1
Figure 1
- With the same partner discuss how Figure 2 can be used to "prove" : x + 1/x
>= 2.
Now partner B should write the "proof" while the other partner (A)
checks to see the writing fully reports their understanding.
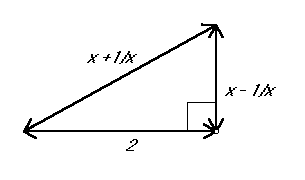 Figure 2
Figure 2
- Friday, August 25 [indicates material of interest that was not discussed in class.]
- Two concepts from
Solow: Unification and Generalization.
- Examples of unification
related solving equations such as 3x=15, 3x=24; then 3x=7 and 3x=N.
- Considered methods to solve these- especially 3x=15.
- guess and check.
- "Guess": x=5.
- Check: 3*5 = 15 . Notice the check here resorts to
common experience and background- as well as understanding of what
constitutes a solution.
- [symbolic recognition of patterns, and ]
- algebraic solution using analysis. - "showing your work"
- consolidated version: x = 3x/3 = 15/3 = 5.
- "step by step" version:
3x = 15
1/3(3x) = 1/3 (15)
x = 5
- "two column analysis"
Statement
|
Reason
|
3x = 15
1/3(3x) = 1/3 (15)
x = 5 |
Given
"Equals multiplied by equals give equals."
1/3(3x) = x; 1/3(15) = 5; "Transitivity of equality."
|
This approach can be more thorough but can still leave out some details.
- Comparison with a calculus problem: Find `int_0^1 x^3 dx`.
- An answer was proposed, 1/4, but there was no comparable way to "check this answer"
- Show the work: (1)`int_0^1 x^3 dx = 1/4x^4 |_0^1 = 1/4 - 0 = 1/4`
Notice that some of this work is notational and the key step involves the "Fundamental Theorem of Calculus."
- Both problems are looking for a number as an answer that
is described in a context, but the answer in the first one has a
context that provides a way to "check", while the integration problem
relies on a "theorem" to justify its correctness.
- Comparison with (2) `int_{-1}^1 1/{ x^2}dx = -1/x |_{-1}^1 = -1 - (-1) = -2` [THIS IS AN ERROR!].
- Consideration of the area interpretation of the definite
integral shows there must be something wrong with (2). The error lies
in the application of the fundamental theorem - the hypothesis
that the function be continuous on a closed and bounded interval is not
satisfied in (2) because `1/{x^2}` is not continuous at `x=0`.
- Consider 3x=7.
- Both a guess and check and analytic approach can work here.
- It now becomes noteworthy that a solution for this problem may not exist if the only type of solution that makes sense is
with natural numbers or integers. The solution here in the context of
common arithmetic needs to have a number called "seven thirds."
- The context of a problem is important in understanding
what an acceptable solution may be. This feature of the problem is
sometimes left unstated or may be made explicit in the statement by
words like "suppose x is a natural number.. " or " if x is any real
number and... "
- Discuss the solution to the unification in Ax=B being solved
by x= B/A.
This follows the analytical process to arrive at a "closed form" solution.
- A generalization of this problem in algebra : Any
(quadratic) polynomial will a root - poosibly involving a complex
number.
- Monday August 28:
- another context to examine Unification and generalization.
- Show: if f(x) = x2 then f '(3) = 6-
- use limits and the definition of the derivative.
- Move directly to
a generalization that f '(a) = 2a.
- demonstrate directly
again using the definition of the derivative and limits.
- Discuss:
one could do similar work to show that if f(x)= x3 then f '(x)
= 3x2
- one could arrive at a unification in the statement:
If n is a natural number and f(x) = xn then
f '(x) = nxn-1.
- If we think of n as a negative integer, say
-4, then this is an example of generalization because the statement of
the result extends to instances which are not covered by the common experience
with 2 and 3.
- Likewise we can genralize the result to f(x) = xr where
r is any fraction or more generally any real number, but a proof
of that result might involve much more complicated arguments.
- Some fundamental discussion of sets....
Introduction to Sets
Representation of Set
Basics(Equality, Subset, Etc)
Optional on-line exercises 1
Set Operations
Optional on-line exercises 2
Properties of Set Operation
- Proofs: Conditionals and "forward backward"
A discussion of a homework
problem related to generalization. The first problem generalize from
a quadratic expression in x to a general real valued function of
x.
The second problem (more difficult) generalized a specific linear inequality
in two variables to a more general linear inequality in two variables,
and then to three variables, and finally to n - variables. Also under this
problem was the relation of (0,0) to the inequality and how that would
be involved in the generalization.
- Some important sets that we will
consider this term are as follows:
| the natural numbers |
N |
{1,2,3,...} or {0,1,2,3,...} |
| the integers |
Z |
{0,1,-1,2,-2, ... } |
| the rational numbers |
Q |
{ n/m where n and m are integers and m not 0} |
| the real numbers |
R |
{ numbers that can be represented as decimals} |
The issue of being a member of a set can be an issue of the nature
of the set or the nature of the object. For example, we can consider sqrt(2)
and ask, "is this a real number?" "Is this a rational number?" To determined
if sqrt(2) is a member of R,we
must have a way to determine this number as a decimal, that is to determine
what digit would be in the kth decimal place for any k. Now this is possible
through some estimaton procedures, though there is no closed form solution
to determine the kth decimal place of the sqrt(2). On the other hand the
sqrt(2) is not a member of the rational numbers. This is not because the
rational numbers have a problem with the definition of that set, but is
a question of mathematics to demonstrate that this number cannot be expressed
as a ratio of integers as required by definition for membership in the
set of rational numbers.
The nature of the subset relation. Focus on the importance of recognizing
the universal set involved in a discussion. Thus for example the set {x:
0 = 10 + 2x + x2} is an empty set if the universe is
the real numbers, but contains two members if the universe is the set of
complex numbers, C.
Some examples
of sets of functions... C0 = { continuous real valued functions
defined on all the real numbers} and C1 = { differentiable real
valued functions defined on all the real numbers}. The issue was whether
these sets are equal.
the subset relation
: when set A is a subset of a set B. The definition of this
term can be made in the form of an absolute statement: "every element of
A is an element of B" or as a conditional statement: " if f is an
element of A then f is an element of B".
Observe that
C1 is a subset of C0. To prove this we suppose
that f is a member of C1and thus f is a differentiable
function. It is a result of the calculus course that if a function is differentiable,
then it is continuous. Thus f is a continuous function, which means
it is a member of C0 .
To consider the related issue, we
ask "Is C0 a subset of C1 ?"
Here the answer is : NO. To show that this is true we examined the function
f
(x)= |x|. This function is continuous for all real numbers,
but is not differentiable at x = 0. This gives an instance where
the conditional requirement for a subset fails, since the hypothesis is
true for this function but the conclusion is false. The example also
shows why the absolute statement is false by giving a single member of
C0 which is not a member of C1 . This lead
to a discussion of the truth or falsity of conditional and universal
absolute statements in mathematics.
How to prove sets are equal using A={ 2, -2}
and B = {x : x2 = 2}.
(i) First, A is a subset of B. This is demonstrated by checking the
equation of B for x = 2 and x = -2.
(ii) Then it is neceassary to show that B is a subset of A. For this
we suppose x is an element of B, so that x2 =
2, and then x2 - 2 = 0, so (x +2)(x-2)
= 0. considering the last equation, together with the fact that if
the product of 2 real numbers is 0, then one of the factors is 0, we see
that either x = -2 or x = 2. In either case, x
is a member of A.
- The discussion focussed again on finding the truth
values for complex statement using conjunction (and), disjunction (or),
conditional (if..then), and negations (not).
We then discussed how simple statements appeared in compound statements
using "and", "or", "if...then", and "not" and how to determine the truth
of the compund sentence from knowing the truth of the simple statements.
We examined wht there were 4 possible ways to have truth values for a pair
of simple statements and how these would deteremine the truth value of
a compund statement using a "truth table."
| A |
B |
A and B
|
If A then B |
|
T
|
T
|
T
|
T
|
|
T
|
F
|
F
|
F
|
|
F
|
T
|
F
|
T
|
|
F
|
F
|
F
|
T
|
This discussion will be continued next class.
-
2/3 The discussion focussed again on finding the truth
values for complex statement using conjunction (and), disjunction (or),
conditional (if..then), and negations (not).
A proposition is called a tautology if it is true
without regard to the truth or falsity of any of its component statement.
A proposition A is said to be equivalent to another proposition
B is the truth value of A is the same as the truth value of B for
any choice of truth values for its primitive components.
We also considered the connective "if and only if", <->, called the
biconditional. It has the following truth table:
| A |
B |
A <->B
|
|
T
|
T
|
T
|
|
T
|
F
|
F
|
|
F
|
T
|
F
|
|
F
|
F
|
T
|
Proposition: The proposition A is equivalent to the proposition
B when and only when the proposition A<->B isa tautology.

