The sum of the measures of the interior angles of a triangle is 180 degrees.
Question:What about a quadrilateral? and a pentagon? or an n sided polygon ( an "n -gon")?
Recall:
* Scissors congruence:
A sc= B means figure A can be cut into pieces that can be reassembled to
form figure B.
This is also described
using the word "equidecomposable". "A and B are equdecomposable to
B."
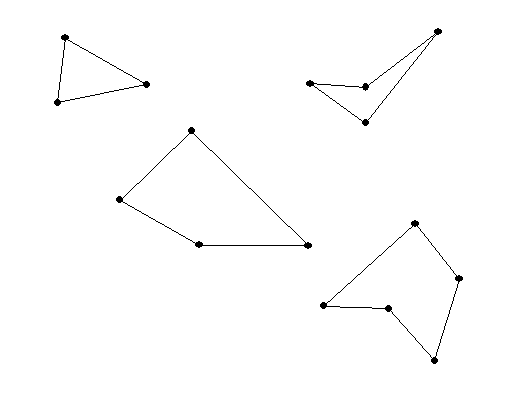
Consider a polygon with n sides.
When n = 3 this is a triangle, n=4, a quadrilateral, or
when n= 5, a pentagon.

The sum of the measures of the
interior angles of a triangle is 180
degrees.
Question:What about a quadrilateral? and a pentagon?
or an n sided polygon ( an "n -gon")?

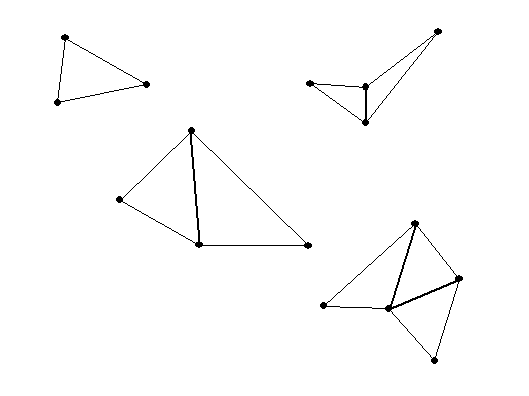
From the figure we can see that for a
quadrilateral (n =4), which can be dissected into two triangles,
the sum is 2*180= 360 degrees.
And for a pentagon (n=5)
which can be dissected into 3 triangles, the sum is 3*180=540
degrees.
A regular polygon is a polygon where the sides are all of equal length and the angles are all congruent (or of equal measure).
For a triangle, the individual angle is 180/
3 = 60 degrees.
For a square, the individual angle is 360 /
4 = 90 degrees.
For a regular pentagon.... 540/5=108
degrees.
Now for a HEXAGON (6 sides) the sum of the angles
is (6-2)* 180= 4*180 = 720 degrees.
So ... for a REGULAR HEXAGON, the individual
angle is 720/6= 120 degrees.