Tuesday, February 24
Comment on Project Proposals and portfolio
sample entries.
Review of Discussion So Far on Classification of Isometries
Video : Isometries
The video introduced the four isometries we have discussed:
reflections, rotations, translations, and glide reflections.
It showed that the product of two reflections is either a rotation (if
the axes of the reflection intersect) or a translation (if the axes
of the reflection are parallel).
We saw this also with a Wingeometry demonstration for reflection-
one and two reflections
he video showed that
Any plane
isometry is either a reflection or the product of two or three
reflections.
Two reflections = rotation or translation.
What about 3 reflections?
Three reflections = reflection or glide reflection
Preview of visual Proof: Key idea- The product of two reflections is "flexible."
How to figure out what isometry you have.... match features.
|
Preserve
Orientation |
Reverse
Orientation |
| No Fixed points |
Translation |
Glide reflection |
| Fixed Point(s) |
Rotation |
Reflection |
Using Isometries to create variations of tilings
Kali:
Symmetry group
180 degree Rotations
Activity for modifying tilings
Translations
Comment on Symmetry
in Music and Sound:
we discussed
Translation .... by an octave, relative position-- chords
Reflection
Rotation: thirteen notes in "chromatic" scale
Glide Reflection
Space: How do we understand objects
in space?
How can the Flatlander experience the sphere and space?
Try making a torus with 2 and 1 piece!
Cross sections: Look at the cube with cross sections : squares,
rectangles, triangles and hexagons depending on how the square passes
through the plane.
Shadows: Look at how the cube might case shadows that were square, rectangular or hexagonal,
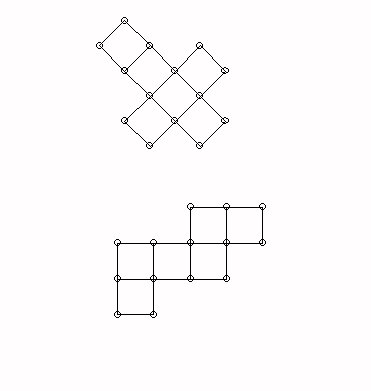
Fold downs- flattened figures: Consider how the cube can be
assembled from folded down squares in two different configurations: a
cross or a "zig-zag."
analogue... point... line.... polygon.... polyhedron......
- Some Issues we'll consider in space:
- Polyhedra and symmetry.
- Historical Note on Kepler.
- Platonic (regular convex polyhedra) and Archimedean
(semiregular convex) Solids- on the
web!
-
-
Why are there only 5?
- Look at the possible ways to put a single
regular polygon together with more of the same to make a spatial "cap"
about a single vertex. This involves equilateral triangles (3,4, or 5),
squares (3) or pentagons (3).
- This shows that there were at most five vertex caps possible. These actually do work to make
- Regular polygons around a vertex.
- All vertices are "the same"
- Activity: Counting vertices, edges, and faces for the platonic solids to become more familiar with them.
- Isometries in space: products of reflections
in space:
- Rotations and translations
- Applications to dance