Thursday,  December 11 : The last class and project fair.

    Presentations of Student Projects.

    Final grades will be submitted by Friday 12-19.
    Projects and portfolios may be recovered on 12-19 or at the beginning of Spring term.

    At the end of class, 3:05 pm, there will be time for a course evaluation.

    Your  comments and suggestions related to Math 103 are welcome.
    You can send them to me directly by E-Mail: flashman@humboldt.edu

    Last class we continued the  discussion of

    Surfaces:
    a sphere
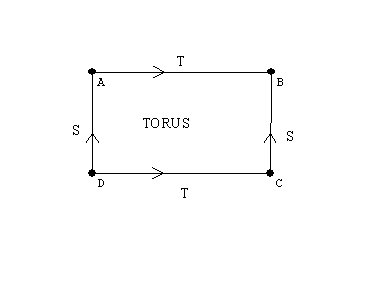
    a torus


  Torus

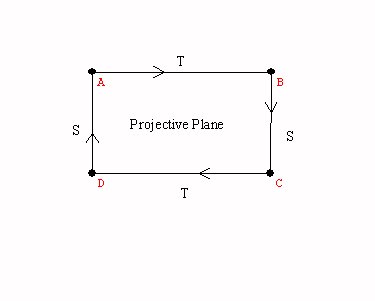
    a Klein bottle


Klein Bottle
,

    the projective plane... The euclidean plane with the horizon line.

    Why is this a closed and bounded surface?
    Response:
    Each point in the ordinary plane corresponds to a point on the upper hemisphere.
    An ideal point on the horizon will correspond to two points on the equator.
    These two points need to be identified to give a one point for one point correspondence of this representation of the projective plane. [download winplot file]



Now flatten the hemisphere down to a disc.
Then we need to identify opposite points on the circle that is the boundary of the disc.
Stretch the disc into the shape of a rectangle.
Now we will need to identify points on opposite sides that have the line connecting them pass through the center of the rectangle. Thus the projective plane is represented by the flattened map---

Boy's Surface ;


    A sphere with a cross cap     Crosscap .


Spheres with handles,


    Visualizations of surfaces by flattened - cut apart models.
    A cylinder, a mobius band, the torus, the Klein bottle, the projective plane.

    Closed Surfaces: Handles and cross-caps  attached to the sphere.
Torus
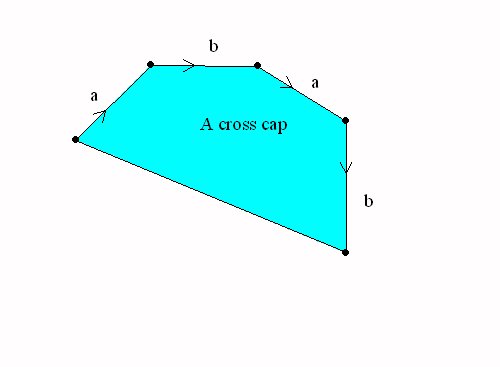
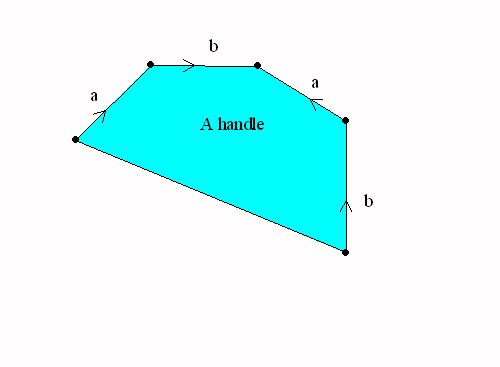
A sphere with a handle = a torus
 Crosscap
A Sphere with a cross cap = the projective plane


    The Topological Classification of "closed surfaces."

    Every connected closed and bounded surface is topologically equivalent to a sphere with handles and crosscaps attached.

    Proof...discussed last class.

Other interest in surfaces: Examples

" If Mathematicians Made Pretzels" Proof without words.
The following two figures are topologically equivalent.
From the Fun Fact files, hosted by the Harvey Mudd College Math Department , 

Unbelievable Unlinking

Figure 1
Figure 1
Figure 2
Figure 2

Imagine that the two objects in Figure 1 are solid (with thickness) and made of very flexible and stretchy rubber.

Question: is it possible to deform one object into the other in a continuous motion (without tearing or cutting)?

Surprise answer: Yes!!

Hint: it is important that the object is solid and has thickness; this transformation cannot be done with a one-dimensional piece of string. It is also not possible to do this with a piece of rope because even though the rope has thickness, it is not flexible or "stretchy" enough. See below for an explanation and animated gif. Or, don't scroll down if you want to think about it a while!

The Math Behind the Fact:
One way to do this is the following. Widen one of the loops and move one of its handles along the stem between the two loops to the other loop and push it through the hole so that the two loops become unlinked. The reference contains a sequence of pictures of this transformation.

Graeme McRae has generously contributed the animated gif in Figure 2, showing another solution to this problem! (Thank you, Graeme!)
Here is the original picture:

Ways to think of surfaces : cross-sections/ projections/moving curves/ using color to see another dimension.   ChromaDepthTM 3D

Generalization of surfaces are called "manifolds".  Cross sections / projections/ moving surfaces-solids.

Transforming surfaces: "turning the sphere inside out." video
             




Thanks!  :)
Final Videos: Flatland and .....?