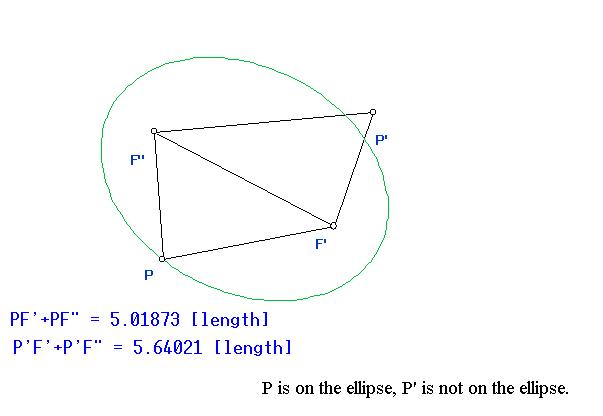
- i....related to 2 foci (ellipse and hyperbola) or 1 focus (parabola),
ii...related to a focus and directrix which was also related to eccentricity.
- Using two spheres tangent at two focii (F1 and F2) to the sectioning
plane that also touch the sides of the cone, the ellipse is characterized
by the distance from a point on the ellipse P having the sum of the distances
PF1 + PF2 a constant,
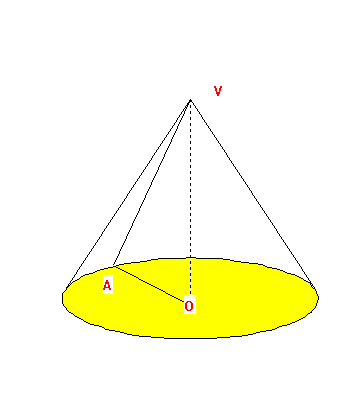
Usually we think of the point lying on the line perpendicular to the center of the circle and only the part of the cone determined by line segments from the vertex and the points on the circle. However, the cone does extend indefinitely beyond the circle and the vertex as the lines extend indefinitely. When we cut a cone with a plane not passing through the vertex we determine a curve in the plane, described as the "conic section." [If the plane passes through the vertex the interesection can be a point (the vertex itself), a single line element of the cone, or a pair of intersecting lines that meet at the vertex.] These are circles, ellipses, parabolas, and hyperbolas.
-
while the hyperbola has the magnitude (absolute value) of the difference
of these lengths |PF1-PF2| a constant.
-
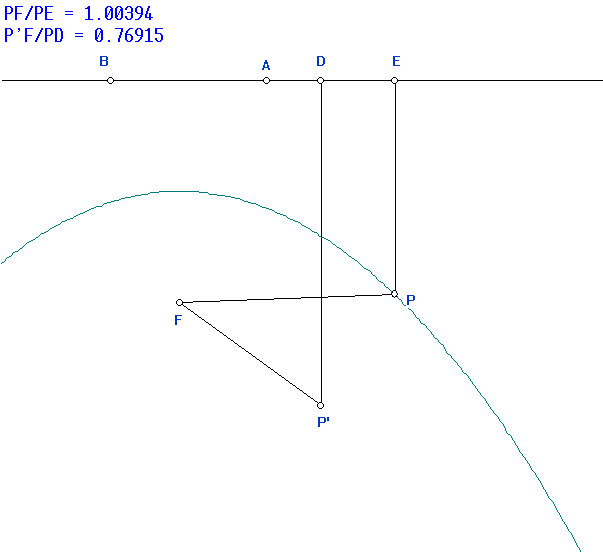
Again using the spheres and choosing one of these, consider the plane through
the sphere at the circle where the sphere touched the cone. This plane
meets the sectioning plane on a line, called the directrix, d. The
film shows that for any conic section a point P on the conic is characterized
by considering the ratio of PF1 to the distance from that point to the
directrix, Pd . This ratio (called the eccentricity of the conic,
e) is a constant for each conic section, in fact the ratio of the sines
of the angle between the sectioning plane and the circle's plane and the
angle between the cone and the circle's plane.
- Notice that from the ideas of projection from the vertex of the cone, any of the conic sections could be the shadow cast by the circle on a sectioning plane.
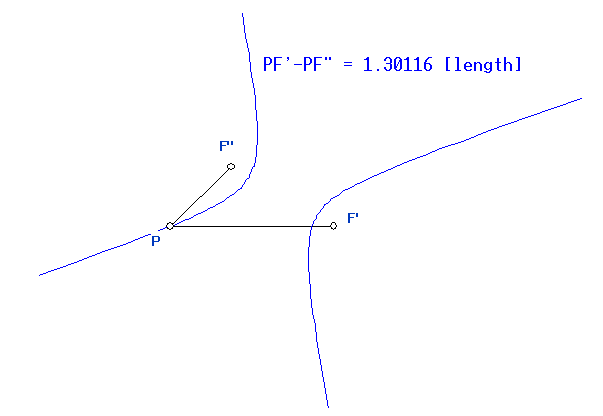
The parabola which in a sense lies between the ellipses and hyperbolae does not fit into these descriptions using two focii. The film explains and visualizes these relations.
The following table summarizes the results related to this ratio. It is fully explained in the video.
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|