Chapter 0.B1 Numbers, Units, Coordinates, and Variables.
Chapter 0.B   Backgrounds
1. Numbers, Units, Coordinates, and Variables.
  © 2002 M. Flashman.
June, 2006: This page now requires Internet Explorer 6+MathPlayer or Mozilla/Firefox/Netscape 7+.

Certainly in your previous experience with mathematics you have encountered a variety of objects which have been described as numbers: counting numbers, whole numbers, integers, fractions, decimal numbers, real numbers, imaginary numbers.  Though we won't start a lengthy philosophical discussion about numbers, here are some questions worth considering initially on our way toward the study of the calculus. "What is a number? How do we decide when something is a number? How do we distinguish  numbers? How do we come to have knowledge about numbers?" Though these questions may seem simple, they are not easy to answer with certainty. In fact, numbers have been the source of much deep and probing discussion by philosophers from Plato and Aristotle to Immanuel Kant and Bertrand Russell and the American philosopher Willard V.O. Quine. Well, if these names don't mean much to you now, you might do a little research on them in the library or on the internet. The point is that the concept of number is worth a little careful thought, not just now, but throughout your encounters with mathematics.

Number Examples: One simple but powerful way to begin to explore a concept informally is to look at examples. So let's recall some of the different kinds of numbers with the notations traditionally used to represent them. First perhaps are the counting numbers: 1,2,3,...These numbers have so many uses that they appear in some form in most cultures where any kind of number distinctions have developed. The notation we use for the counting numbers developed using Hindu-Arabic numerals brought to Western Europe in about the 12th century. Some authors use the term natural number to describe the counting numbers. We will follow the more common convention of mathematics today and use natural number  to describe the counting numbers together with the number 0. We'll denote the set of natural numbers with the letter N, so that N = { 0, 1, 2,... }. The non-zero natural numbers are the counting numbers which are also described as the positive natural numbers and are denoted N+.

Integers: To make sense out of contexts where numbers are used to represent changes in quantity, the opposite of counting numbers, called negative numbers, were developed. These numbers, -1, -2, -3, ... , can be considered as solutions to very simple problems such as "what number added to 5 will give a result of 2 ?" Of course, the answer, developed to answer this question, is the negative number -3, because 5 + (-3) = 2. The integers, denoted Z, consist of the natural numbers together with their opposites, i.e., the numbers 0,1,-1,2,-2,3,-3,... . So we have another way to describe the counting numbers, this time as the positive integers, denoted Z+. We will use a letter usually from the list i, j, k, l, m, n, p, q when we want a symbol to represent an unknown or arbitrary integer (or a natural number)

The issues surrounding what constitutes a number are too numerous to list here, but here are a few to whet your appetite:
  • Are numbers a result of experience? 
  • Are numbers a result of convention? 
  • What is the relation of numbers to numerals (symbols)? 
  • What is the relation of numbers to their uses? 
  • Are numbers always components of some structural system? 
  • How do numbers work as part of a language for science? 
  • Can numbers always be related to geometry? 
  • Are there some key properties (axioms) of numbers  which logically determine all their other properties? 

    • You can learn more about these by reading The Mathematical Experience by P. Davis and R. Hersh. 

     
     
    Rational Numbers: Another way to develop more number examples easily is through comparisons based on the concept of ratio. Comparing counting numbers with ratios is described in grade schools as the study of fractions. Numbers like 2/3, 4/7, 6/8, ... are all of this type. The arithmetic of these numbers is a little more subtle than that of the integers and even the notion of equality takes a little more care. Even though the numbers don't look alike, 3/5 is said to be equal as a number to 24/40. Like negative numbers, these numbers can also be considered as solutions to very simple problems such as "what number multiplied by 5 will give a result of 3?" Of course, the answer, developed for this problem, is the number (ratio) 3/5, because 5 * (3/5) = 3. There are positive fractions (ratios of whole numbers) while the opposite of a positive fraction is a negative fraction, such as -3/5. Fractions, or rational numbers, are denoted by  k/d or `k/d` , with k being called the numerator (how many) and d being called the denominator (what kind). The numbers k and d can be any integers as long as the denominator, d, is not zero. The collection of all fractions is called the set of rational numbers and this set is denoted Q. As you might guess, the set of positive rational numbers is denoted Q+ .
     
    Irrational Numbers: We certainly have not exhausted the examples of numbers that you have encountered. Many numbers were first recognized in studying algebra and geometry. One such number is the square root of 2, denoted  `sqrt{2}`. This number illustrates some typical difficulties in the concept of number. As with the rational numbers, this number does solve and algebra problem. We can think of  `sqrt{2}` is the solution to the problem, "what number has its square equal to 2?"  The difficulty with this number is that `sqrt{2}` is not a rational number. [The end of this section has an argument that justifies this last statement.] So is it a number at all? and if so, what kind of number is it? Fortunately, this number has a geometric interpretation as well, namely, it is the ratio of the length of a square's diagonal to the length of its side. See Figure 1. So at least `sqrt{2}` seems to have meaning through its geometric interpretation. If a square has a side of length 1, then its diagonal will have length `sqrt{2}`. Thus it does make sense to think there is such a number. We will say more about `sqrt{2}` later.  
    Figure 1
     
    Another example of a number that is not rational is  `pi`.[For a history of the number `pi`, see *** ] This number also has a geometric foundation, being characterized primarily by its interpretation as the ratio of the circumference of any circle to its diameter. In fact, `pi` is quite unlike `sqrt{2}` in its algebraic character. Ferdinand Lindemann (1852-1939) demonstrated in 1882 that  `pi`,  is not the solution to any simply stated algebraic problem involving the usual arithmetic operations and the integers. Numbers that fail to be the solution to "simple" algebraic problems are called transcendental numbers. [More precisely: A number is called algebraic if it is the solution to an equation that is described using only the integers and the simple operations of arithmetic. A real number that is not algebraic is called transcendental.]
    Decimals:  The development of notations for numbers and the science of measurement have also contributed much to the extension of number concepts. In about 1585 Simon Stevin  proposed decimal notation as a tool for expressing and manipulating all numbers. Later, after the work of René Descartes (1596-1650), much of geometry in the seventeenth and eighteenth centuries was reduced to the study of numbers. Thus the concept of a real number developed through the centuries to include all numbers that could represent measurements of length based on the choice of some unit for measuring or could be estimated to any decimal precision with a decimal number. School children work with these notions beginning with manipulatives such as cuisenaire rods. The continued debates over the acceptance of the metric system in the United States demonstrate the importance of decimals in the choice of units for even the most common of daily experiences. How do we know we have an accurate decimal representation for `sqrt{2}` or `pi`? Even such rational numbers as 3/7 require an infinite process when described in decimal notation. A key fact is that any rational number when expressed in decimal notation will involve a block of digits that will repeat indefinitely in its decimal representation. But unlike 3/7 and other rational numbers, the decimal representation of any number that is not a rational number will not have a repeating block of digits.
    So how do we tell what digit is in each position of the decimal expression for `sqrt{2}` or `pi`? In fact we don't have a method for knowing these in any precise formulation comparable to the way we can describe the decimal expression for 3/7.  The development of procedures to obtain better descriptions of the decimal expressions for these and other numbers has been an important and lengthy part of mathematical history. To shorten our discussion of numbers and their history, we'll stop here on the trail.
    In summary, the numbers that can expressed with the (possibly infinite) decimal notation, and can be interpreted as the measurement of a length using some fixed unit, are called real numbers. We will denote the set of real numbers with the symbol  (and the positive real numbers are denoted Â+). Notation: When we want a symbol to represent a real number  we will usually use one of the letters a, b, c, p, q, r, s, t, u, v, w, x, y,or z. Often we will want to consider a real number that measures a change or difference between other numbers. In this case we will often denote the real number by the letters h or k, or symbols like `Delta x` or `Delta y`. [The  symbol `Delta` is the Greek letter "delta." It is often used in mathematical notation to indicate a change, as in this case, where it indicates the change in the quantity measured with the variables x or y.]
    Imaginary and Complex Numbers: The examples of numbers do not stop with the real numbers. As you may recall the algebraic properties of the real numbers and the operations of addition, subtraction, multiplication, and division together with their ordering properties imply that the square of any real number is not a negative number. Thus a solution for the algebraic problem of finding a number which has its square equal to -1 is possible only if the solution is not a real number. The number `i=sqrt{-1}` is the solution to this problem. Numbers of this type are not real numbers, and are called imaginary numbers. The algebraic properties of imaginary numbers are sometimes a little troublesome, but they too serve some very useful purposes and can be visualized with a little effort as part of a larger collection of numbers that include such examples as 3 + 5 i and  `2-sqrt{3}i`. These combinations of real and imaginary numbers are called complex numbers, with the set of complex numbers denoted by C. The complex numbers have a very important role to play in practically every aspect of mathematics today and in fact has its own part of the calculus. Our work in this beginning of the calculus will focus primarily on real numbers and contexts where the real numbers are adequate for a description of the relevant measurements.
    Units and Measurement: The use of units in measuring everything from distances between objects to the forces that act upon them to the duration of time is so common place in our lives that we often overlook how these units are determined and adopted as conventions to facilitate communication. New units develop and older ones fall into disuse. The need to make changes of scale between units is a consequence of the variety of units as well as the utility of having units for describing features at different levels of discourse. Inches and centimeters may be appropriate to measure computer discs or keyholes, but light years are much better for measuring the distances between stars. Grams and ounces are appropriate for measuring sugar in a cake recipe, but not for measuring the mass or weight of a dump truck or an elephant. Dollars and cents seem appropriate for measuring the cost of a meal at a restaurant, but not for measuring the budget of the United States federal government or the World Health Organization. Once a unit is established, then numbers can be interpreted in terms of that unit of measurement. Each interpretation through the unit gives a number  meaning in its application  beyond the abstract number itself. 5 inches, 5 centimeters, 5 light years, 5 grams, 5 ounces, 5 tons, 5 dollars, 5 cents, 5 trillion dollars,.... The connection between numbers and measuring units is one of the crucial aspects of the application of mathematics to real contexts. Try not to lose sight of this connection even when we focus on the more abstract aspects of the mathematical concepts. The interaction of mathematics with interpretations and applications is subtle and not fully understood in all its philosophical and practical details, so feel free to question these things. In your struggles to understand numbers and their uses you will  arrive eventually at your own view that will allow you to make sense of this subtle connection.
    The Number Line: Let's return to our subject, more precisely, the use of units to interpret numbers. One of the chief interpretations of real numbers is in geometry as the measures of distances. Once a unit of distance measurement is established along with a point and a direction on a line, we can establish a correspondence between the points on that line and the real numbers. This correspondence is frequently confused so that the numbers are considered to be the points on the line. Let's be very clear about this. The distinguished point on the line that corresponds to the number 0 is not the number 0. That point can used to visualize the number 0, it can be interpreted as the number 0, it can even be labeled with the numeral 0, i.e., the symbol we commonly use to represent the number 0, but it is not the number 0. Of course, 0 is called the coordinate of that point. Similarly, any point on the line could be used, interpreted, and even labeled with an appropriate number based on the chosen unit, point and direction, but the point is not the number. Thus `sqrt{2}` and `pi` will be the coordinates of two distinct points on the line. This may belabor the issue, but the issue is important.
    Numbers are mathematical objects that can be interpreted in many contexts. In the geometrical context of the number line, numbers can be interpreted as points and are called the coordinates of points. The issue of distinguishing a mathematical object from its interpretations and applications is worth making sense of here. It recurs often in the development of the mathematics and recognizing this distinction can help make sense of other, sometimes unclear, uses of language.
    Summary: Try to distinguish mathematical objects from their interpretations.
     

    Mathematics is sometimes described as the language of science. The language aspect of mathematics involves notation, naming, and concept definition. Many features of a language are organized by historical accident and use, following precedents that seem convenient for the purpose of communication. Other aspects are consciously determined through open discussion of the value of certain choices to the purposes of the language. Whether by choice or history, the language of mathematics is dominated by conventions. You can see this conventional quality clearly in the symbols and names we give to numbers. It is something you should note occasionally as we proceed that we develop (mathematical) concepts through experience, motivation, examples, and definitions that name objects, properties, and relations. Moreover we introduce notation for these concepts to organize and communicate our knowledge, making further investigations and applications sensible. The notation of mathematics has a long and illuminating history, connected to the development of the mathematical concepts and their applications.
     
    For example, we can look at the concept of a multiplicative inverse. We say that 1/3 is the multiplicative inverse of 3, using the words to describe a relation between the numbers 1/3 and 3, while we can discuss the multiplicative inverse of the number `pi`, referring to a number described by its relation to the number  `pi`. The words "multiplicative inverse" name a relation or a number, so the designation is ambiguous without establishing a context.  Looking at the number use of the words, we can articulate a definition of words to describe a number. So we might say that a number a will be called a multiplicative inverse for a number b if the product of the two numbers, ab, is 1. We have a notation for this as well. We write `1/b=b^{-1}` to denote a number that is a multiplicative inverse for b. 

    Now to describe a number does not necessarily mean that such a number exists! We can talk about a multiplicative inverse for almost all numbers and make sense of how that number might be described in some other fashion that would allow us to acknowledge its existence. However, using conventional arithmetic for real or complex numbers, there cannot be any multiplicative inverse for 0. The reason is that the result of multiplying any number by 0 is 0, and 0 is not 1. So no number can satisfy the defining condition for it to be a multiplicative inverse for 0. You might consider other situations like `1/0` where an object or concept is defined by describing conditions under which it would be appropriate to use the term but where no actual object exists. This may happen even when a notation apparently indicates an object. In a way, mathematicians are like creative writers who can describe and name fictitious animals called unicorns when no such animal exists in our world.
    The ambiguity of notation: What does "-" mean? When you see an expression involving the minus sign, it can mean different things depending on the context. When discussing numbers the notation -5 indicates we are discussing the number "negative 5" or the number which when added to 5 would give 0 as a result. Some might even distinguish this use of the minus sign by writing it slightly higher than the numeral 5, as -5. A second  meaning for the minus sign is to indicate the opposite of the number  that follows the sign. This can be a little confusing, since writing -5 indicates both a negative number and the number that is the opposite (or additive inverse) of 5, but the use in this sense is easier to identify when we write an expression like -(-5) describing the number 5 which is the opposite of -5. Finally, the minus sign is used to indicate the operation of subtraction in an expression such as 5-3 which is another way to denote the resulting number 2. Of course there are situations where all three interpretations can come into play, as in the expression 10-( -5) which  can be found by computing 10-( -5) = 10 + 5 = 15..
       
    Variables, Constants and Parameters: Using a symbol to represent a number or any other mathematical object is one of the keys to the power of mathematics. We have established by convention notations for numbers that allow us to designate a number by a symbol or a string of symbols. Once we recognize the distinction between the symbols and the numbers they represent, we can begin to understand notation being used to represent a number (or other mathematical object) that is not known. Perhaps the represented number is characterized by being the solution of an equation. Perhaps it is a number that corresponds to a measurement which has not or cannot be made. The meaning or designation of the symbol can change or vary to suit a context or may be entirely determined by conditions we may or may not know. It can even be that the symbol is being used when there is no number that it could possibly represent. This last situation may be like a discussion of unicorns- these animals do not exist- yet we can describe them as if they did exist! The symbol can represent a quantity determined by many known and unknown influences. In other words, the quantity's numerical value can  vary, and so the symbol is called a variable[1] representing a changeable quantity.
     
    The actual use of a symbol to represent a number can depend on the context. At times a symbol is meant to represent a single number that will remain the same throughout a discussion. For instance we let a denote my current age measured in years and A denote the amount of money I invested in  stocks on January 1, 1996 measured in dollars. These symbols are called constants because the quantities they represent do not change in the context. Letters commonly used for constants are a, b, c, d, and k. At times a symbol represents a number that will depend on the context, and hence be variable. Yet once the context has been determined the number will remain constant. We previously let a denote my age measured in years and A the amount of money I invested measured in dollars. Now these symbols represent numbers that can vary depending on the specification of more information about the context. My age depends on the date at which it is measured while the amount of my investment  depends on the time and the form of investment (stocks, bonds, or a savings account). So these variables can turn out to be constants depending on the specification of a context. The word parameter[2] is sometimes used to describe a symbol used to represent a quantity that is variable yet in some investigations will be considered as a constant determined by the context. The variable t when used to represent time is often considered a parameter since we often want to examine the relation of quantities at a single moment in time, thinking of perhaps taking a snapshot to record that moment, and thus holding time constant.
     
    Operations, Equalities, and Inequalities: It goes without saying that much of the mathematics you have studied has dealt with the arithmetic operations of addition, subtraction, multiplication, and division and the processes related to determining numbers that will satisfy relations determined by equations. You will have some review exercises at the end of this section to check yourself on these skills, including expanding and factoring polynomials as well as solving linear and quadratic equations and inequalities. What you may not have considered at length before is the meaning of these features of mathematics. What does the statement 2+3=5 mean? Can you explain why `(-2)^4 > 0`?
    Equality as identity of numbers: Let's look first at 2+3=5. The symbol "=" placed between symbols representing numbers indicates that the symbols are representing the same number.  The idea is that a single object can be designated in more than one way. You have your name and your social security number. Usually (but not always) if we look at the names on a class list there will be only person represented by that name, and the same is true for the social security numbers on the list. So we might say that Alice Callahan = 396 23 4583 means that these symbols designate the same person. On the left side of the equation the symbol "2+3" represents a single number which results by adding the numbers 2 and 3. On the right hand side we have a number designated by the numeral "5." The assertion of the equation is that these symbols represent the same number. This interpretation works just as well to explain `2+3=35/7`. Both statements are considered true because the symbols on each side of the equations represent the same number. On the other hand the statement 2+3=7 is false because the symbols on both sides  of the equation do not represent the same number, though it may not always be clear how  we know this.
    Equality as defining notation: When we write "let a be the number with the property that `a^3 = 5` " or "`a=root(3)5`" the equality sign might be replaced with º which is sometimes used to reinforce the fact that this use is not asserting an identity but is establishing a representation. In this case the symbol a is being assigned to represent any one or more objects that satisfy the criterion that when cubed the result is 5. Such an object is also represented by `root(3)5`. The equation is merely establishing  another notational representation.
     
    Equality of expressions involving variables: The equation x+5=7 may be true or false  depending on the interpretation of the variable  x as a specific number. If x is 2 then the equation is true, but if x = 3 (or any other number different from 2) then the equation is false. But what does the equation mean when we don't have an interpretation specified for x? An equation with a variable that does not have a specified value is called an open equation. An open equation  is neither true nor false until the interpretation of all the variables have been specified. Such an equation serves as a symbolic form designating a relation between numbers when the variables are specified. Generally these open equations arise as constraints characterizing a number or numbers and the problem is to determine any and all numbers that will make the equation true, i.e., solve the equation.
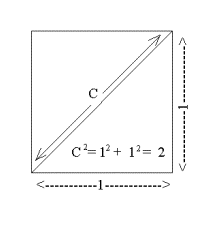
    Inequalities are similar to equations in their meaning. When we describe those real numbers whose squares are less than 25 we have designated a set of numbers  which contains many possible members. In fact it contains any and all real numbers larger than -5 and smaller than 5. That seems easy enough to describe, but the issue can be more subtle when we describe those rational numbers whose squares are less than 2. In this case the set is a collection of rational numbers which seems controlled by an irrational number,`sqrt{2}`.
    The solutions to inequalities sometimes can be expressed as intervals of numbers, such as the positive real numbers less than 5. This interval is denoted (0,5). Recall other examples of notation for intervals from the following:
     
    `[2,5]={x:2<=x<=5}` `[2,5)={x:2<=x<5}`
    `(2,5]={x:2<x<=5}` `(2,5)={x:2<x<5}`
    `[2,oo]={x:2<=x}` `(2,oo]={x:2<x}`
    `(-oo,5]={x: x<=5}` `(-oo,5)={x: x<5}`
     
    `sqrt{2}` is not a rational number. As promised earlier in this section, here is an argument showing that `sqrt{2}` is not a rational number. The argument proceeds by assuming that `sqrt{2}` is a rational number and then demonstrating  as a result of this assumption that something absurd and obviously false would also be true.
    (This type of argument is described as an indirect argument or an argument by reduction to the absurd, reductio ad absurdum) The absurd result with which we will end the argument will be in finding a number that is both even and odd.
    We assume `sqrt{2}` is a rational number. In fact we assume that`sqrt{2}= j/k ` where j and k are integers.  Now the defining property for `sqrt{2}` is that when squared, this number is 2. So it must be that `(j/k)^2 = {j^2}/{k^2}=2` and therefore ` j^2 =2 k^2`.  Now if we consider `j` and `k` factored completely into products of integers and then squaring those factors, we see that `j^2` and `k^2`each must have an even number of 2's for factors (remember 0 is an even number). So the left hand side of the equation ` j^2 =2 k^2` has an even number of 2's for factors, while the right hand side of the equation has one more 2 than the even number of 2's appearing as factors of `k^2`, so that we must conclude by counting the factors that are 2's that some even number is also an odd number. This ends the argument, since it is absurd for an integer to be both even and odd.
    Review Exercises: The exercises for this section cover material  from previous course work in algebra. You may not recall all of these topics or how to do the problems precisely. You  should refer to the texts or notes from your previous courses in mathematics if you find these difficult. The skills and concepts needed to solve these problems will be important in the work ahead- so be careful to identify any difficulties you have with these problems and try to remedy any misunderstandings as you proceed. 
    Rational Numbers and Repeating Decimals: It is common to represent fractions using decimal notation. For instance 1/2 = .5 and 1/4 = .25. More interesting examples require the use of decimals that continue indefinitely, such as 1/3 = .3333.... or 1/6 = .16666... which indicate that the last digit continues to repeated in the succeeding decimal places.
    1. Repeating decimals and rational numbers:
      1. Find decimal representations for the numbers 2/9,  3/7,  3/11 , and 3/13
      2. The number that is expressed by the repeating decimal .38383838.... is a common fraction. Here's how to see that algebraically. Let  N=.38383838.... so that 100 N = 38.383838.... . Now subtract N from 100 N to see that 100N - N = 99N = 38. and so N = 38/ 99.
        3. Study this example, then express the following repeating decimals as fractions: .535353....  ,  5.727272....    ,  37.56838383....
      3. Based on part a explain why .101001000100001.... is not a common fraction.
      4. Based on part b and the text's discussion of `sqrt{2}`, explain why `sqrt{2}`can not represented by a repeating fraction.
    2. Review the argument that `sqrt{2}` is not a rational number.
      1. Replace each occurrence of the number 2 by a 3 in the argument to show that `sqrt{3}` is also not a rational number.
      2. Prove that `sqrt{5}` is not a rational number.
      3. Discuss why this procedure fails to show that 2 =`sqrt{4}` is not a rational number.
    3. Simplify- express as an algebraic expression using fewer operations to evaluate for any value of x that makes sense.
      1. `{x^2-9}/{x+3}`            
      2. `{x^2+6x+9}/{x+3}`            
      3. `{x^2-2x-15}/{x+3}`           
      4. `sqrt{x^2+6x+9}
    4. Rationalize the denominator.    a. `{2+sqrt{3}}/{2-sqrt{3}}`                   b.  `{2+sqrt{h}}/{2-sqrt{h}}` 
    5. Rationalize the numerator.   a. `{sqrt{x}-sqrt{3}}/{x-3}`                   b.  `{sqrt{3+h}-sqrt{3}}/h` 
    6. Determine for which numbers the expression fails to represent a real number.
      a. `{x^2-9}/{x+3}`              b. `sqrt{x+9}`                   c.`{2+sqrt{h}}/{2-sqrt{h}}`                      d.`{sqrt{3+h}-sqrt{3}}/h` 

    7. Solve the following equations: Find any and all real numbers which the variable can represent and have the equation be true.
    a. `2x-5=x+3`        b. `x^2-2x-5=x+3`     c. `x-3=sqrt{x+3}`                d. `5/{x+3}+4/x=3`       e. `x^2+9=4`
    8.Solve the following inequalities: Find any and all numbers which the variable can represent and have the inequality be true. When possible, express the solution in interval notation:
    a. `2x-5<=x+3`        b. `x^2-2x-5<x+3`     c. `x^2-2x-5>=x+3`                d. `5/{x+3}+4/x>=3`
    9.Recall that |a| represents the magnitude or absolute value of the number a. Thus |a| is a when a is not negative and -a when a is negative. For example |5| = 5, while |-3| = 3. Solve the following equations and inequalities that are expressed using the absolute value. When possible, express the solution in interval notation:
    a. `{|4-x|}/ (4-x) = -1`    b. `{|4-x|}/ (4-x) = 1`  c.` |x-10000|<1`.  d.`|4-x| > 5`  e. `|3x - 6| < 9`

    10.Write a short story about a car trip. Discuss briefly those aspects of the trip that might be measured and the units and approximate size of the numbers that would arise from those measurements.

    11.Write a short story about cooking dinner. Discuss briefly those aspects of the meal's preparation that might be measured and the units and approximate size of the numbers that would arise from those measurements.

    12.Write a short story about going shopping. Discuss briefly those aspects of the shopping that might be measured and the units and approximate size of the numbers that would arise from those measurements.

    13.Write a short description of an ecological system. Discuss briefly those aspects of the system that might be measured and the units and approximate size of the numbers that would arise from those measurements.

    14.Write a short description of the human body. Discuss briefly those aspects of the body that might be measured and the units and approximate size of the numbers that would arise from those measurements.

    15.Write a short story about an athletic event or sports competition. Discuss briefly those aspects of the story that might be measured and the units and approximate size of the numbers that would arise from those measurements.

    16.How Ben proved he was the pope.

    Ben arrived yesterday smiling quite broadly.  "I can show you that I am the Pope," he declared with a laugh. "You see I and the pope are two persons. But I can show that 1=2, so I and the pope are 1 person. Thus I am the Pope." But you may ask, "How did Ben show that 1=2?" Here's the  argument Ben gave me.

    "Suppose x=y. Then xy = yy, so xx-xy = xx - yy. Now factoring we see that x(x-y) = ( x+y) (x-y). Now canceling the common factor of (x-y) we have x = x+y.  But we assumed x=y, so x+y = x + x = 2x and 1x =2x. Finally we can surely cancel the number x and we have 1=2. I rest my case! "   What is wrong with Ben's argument? 
    [1] var$i$$able           adj. 1. a . Likely to change or vary; subject to variation; changeable. b . Inconstant; fickle.
    Biology                    2.  Tending to deviate, as from a normal or recognized type; aberrant
    Mathematics            3.  Having no fixed quantitative value.
    n.             1. Something that varies or is prone to variation.
    Astronomy                2.  A variable star.
    Mathematics            3. a. A quantity capable of assuming any of a set of values

    b. A symbol representing such a quantity. For example, in the expression 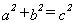, a, b, and c are variables.

    [2]parameter n. 1. Mathematics a . A constant in an equation that varies in other equations of the same general form, especially such a constant in the equation of a curve or surface that can be varied to represent a family of curves or surfaces.
    b . One of a set of independent variables that express the coordinates of a point.
    2. a . One of a set of measurable factors, such as temperature and pressure, that define a system and determine its behavior and are varied in an experiment.
    b.  A factor that restricts what is possible or what results: All the parameters of shelter where people will live, what mode of housing they will choose, and how they will pay for it. New York
    c . A factor that determines a range of variations; a boundary: an experimental school that keeps expanding the parameters of its curriculum.
    Statistics 3.  A quantity, such as a mean, that is calculated from data and describes a population.

    4.  A distinguishing characteristic or feature.