- If we have any two regions on the board of equal area then the dart would land in those regions about the same number of times with a large number of throws.
where it lands on the board. This is our basic experiment. For each of
the following questions include a discussion of how you arrived at your
answer with your response.
(a) If the board is divided into two regions by a concentric circle
of radius 30 and I repeat the experiment a large number of times, what
proportion of markings from the experiment is likely to lie in the inner
region? See Figure 1 .
(b) If the board is divided into four regions by concentric circles
of radius 15, 30, and 45 cm and I repeat the experiment a large number
of times, what proportion of the markings from the experiments is likely
to lie in each of the regions?
(c) If the board is divided into n regions by concentric circles of
radius k * 60/n cms with k = 1,2 ... n-1, and I repeat the experiment
a large number of times, what proportion of the markings from the experiments
is likely in each of the regions? 
(d) What proportion of the markings from repeating the experiment is
likely for the dart to fall within A cms of the center where 0<A<60?
2. Darts and Averages. Consider the same circular magnetic dart board with radius 60 centimeters. Draw 5 concentric circles on the board with radii 10,20,30,40, and 50 creating 6 regions (one disc and five bands). Consider the following game: Throw the dart at the board and score 5 points for landing in the inner disc, 15 points for landing in the smallest band (between the circles of radius 10 and 20), 25 points for landing in the next smallest band (between the circles of radius 20 and 30), 35 points for landing in the band between the circles of radius 30 and 40, 45 points for landing in the band between the circles of radius 40 and 50, and 55 points for landing in the outermost band (between the circle of radius 50 and the edge of the dart board). [In this game a low score is considered evidence of greater skill.]
We allow a player to throw a dart 36 times and find the total score
for the player as well as the average score (the total divided by 36).
Notice the average will be a number between 5 and 55.
Give a total score and an average that are likely for a player with
no special skill. [These numbers are described as the expected score and
the expected average for the game.]
Discuss your reasoning and show the work leading to your proposed solution.
Suggestion: You might investigate the similar problem with 2
regions and 4 throws and then 4 regions and 16 throws as a way to begin
thinking about the problem. How many darts do you think would fall in each
region?
Extension: (Optional) If we measure the distance each of the
36 darts fall from the center, what do you think the average distance would
be for a player with no special skills. Explain your reasoning, any
connections with the expected average score in the game, and whether you
have any belief about the accuracy of your response. (Is it an underestimate
or an overestimate?)
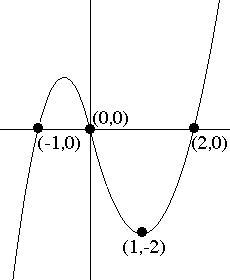
A. Use two curves defined by quadratic functions to make a single smooth curve that passes through the four points, (-1,0), (0,0), (1,-2) and (2,0) as in the figure. Discuss briefly the strategy you used to find your solution.
Suggestion: Let f(x) = Ax2 + Bx + C and g(x) = Ex2 + Fx + G . Have the pair of curves meet at (0,0).
B. Find a second pair of quadratic polynomials which can be used to
make another single smooth curve that passes through the same four points.
Suggestion: Do this similarly to part A but have the pair of curves
meet at (1,-2)
C. Use some technology to sketch your solutions for A and B. Discuss briefly any noticeable differences (such as "vertices") between the three curves.